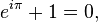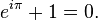Euler's identity

From Wikipedia, the free encyclopedia

Jump to: navigation, search
For other uses, see List of topics named after Leonhard Euler.
The exponential function ez can be defined as the limit of (1 + z/N)N, as N approaches infinity, and thus eiπ is the limit of (1 + iπ/N)N. In this animation N takes various increasing values from 1 to 100. The computation of (1 + iπ/N)N is displayed as the combined effect of N repeated multiplications in the complex plane, with the final point being the actual value of (1 + iπ/N)N. It can be seen that as N gets larger (1 + iπ/N)N approaches a limit of −1.

  Part of a series of articles on
The mathematical constant, e

Natural logarithm

Applications in: compound interest · Euler's identity & Euler's formula  · half-lives & exponential growth/decay

Defining e: proof that e is irrational  · representations of e · Lindemann–Weierstrass theorem

People John Napier  · Leonhard Euler

Schanuel's conjecture

In mathematical analysis, Euler's identity, named after Leonhard Euler, is the equation

e^{i \pi} + 1 = 0, \,\!

where

e\,\! is Euler's number, the base of the natural logarithm,
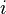i\,\! is the imaginary unit, one of the two complex numbers whose square is negative one (the other is -i\,\!), and
\pi\,\! is pi, the ratio of the circumference of a circle to its diameter.

Euler's identity is also sometimes called Euler's equation.

Contents

[edit] Nature of the identity

Euler's identity is considered by many to be remarkable for its mathematical beauty. Three basic arithmetic operations occur exactly once each: addition, multiplication, and exponentiation. The identity also links five fundamental mathematical constants:

Furthermore, in mathematical analysis, equations are commonly written with zero on one side.

[edit] Perceptions of the identity

A reader poll conducted by Mathematical Intelligencer named the identity as the most beautiful theorem in mathematics.[1] Another reader poll conducted by Physics World in 2004 named Euler's identity the "greatest equation ever", together with Maxwell's equations.[2]

The book Dr. Euler's Fabulous Formula [2006], by Paul Nahin (Professor Emeritus at the University of New Hampshire), is devoted to Euler's identity; it is 400 pages long. The book states that the identity sets "the gold standard for mathematical beauty."[3]

Constance Reid claimed that Euler's identity was "the most famous formula in all mathematics."[4]

Gauss is reported to have commented that if this formula was not immediately apparent to a student on being told it, the student would never be a first-class mathematician.[5]

After proving the identity in a lecture, Benjamin Peirce, a noted nineteenth century mathematician and Harvard professor, said, "It is absolutely paradoxical; we cannot understand it, and we don't know what it means, but we have proved it, and therefore we know it must be the truth." [6]

Stanford mathematics professor Keith Devlin says, "Like a Shakespearean sonnet that captures the very essence of love, or a painting that brings out the beauty of the human form that is far more than just skin deep, Euler's equation reaches down into the very depths of existence."[7]

[edit] Derivation

Euler's formula for a general angle.

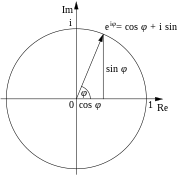
The identity is a special case of Euler's formula from complex analysis, which states that

e^{ix} = \cos x + i \sin x \,\!

for any real number x. (Note that the arguments to the trigonometric functions sin and cos are taken to be in radians.) In particular,

e^{i \pi} = \cos \pi + i \sin \pi.\,\!

Since

\cos \pi = -1 \, \!

and

\sin \pi = 0,\,\!

it follows that

e^{i \pi} = -1,\,\!

which gives the identity

e^{i \pi} +1 = 0.\,\!

[edit] Generalization

Euler's identity is a special case of the more general identity that the nth roots of unity, for n > 1, add up to 0:

\sum_{k=0}^{n-1} e^{2 \pi i k/n} = 0 .

Euler's identity is the case where n = 2.

[edit] Attribution

While Euler wrote about his formula relating e to cos and sin terms, there is no known record of Euler actually stating or deriving the simplified identity equation itself; moreover, the formula was likely known before Euler.[8] (If so, then this would be an example of Stigler's law of eponymy.) Thus, the question of whether or not the identity should be attributed to Euler is unanswered.

[edit] See also

[edit] Notes

  1. ^ Nahin, 2006, p.2–3 (poll published in summer 1990 issue).
  2. ^ Crease, 2004.
  3. ^ Cited in Crease, 2007.
  4. ^ Reid.
  5. ^ Derbyshire p.210.
  6. ^ Maor p.160 and Kasner & Newman p.103–104.
  7. ^ Nahin, 2006, p.1.
  8. ^ Sandifer.

[edit] References

  • Crease, Robert P., "The greatest equations ever", PhysicsWeb, October 2004.
  • Crease, Robert P. "Equations as icons," PhysicsWeb, March 2007.
  • Derbyshire, J. Prime Obsession: Bernhard Riemann and the Greatest Unsolved Problem in Mathematics (New York: Penguin, 2004).
  • Kasner, E., and Newman, J., Mathematics and the Imagination (Bell and Sons, 1949).
  • Maor, Eli, e: The Story of a number (Princeton University Press, 1998), ISBN 0-691-05854-7
  • Nahin, Paul J., Dr. Euler's Fabulous Formula: Cures Many Mathematical Ills (Princeton University Press, 2006), ISBN 978-0691118222
  • Reid, Constance, From Zero to Infinity (Mathematical Association of America, various editions).
  • Sandifer, Ed, "Euler's Greatest Hits", MAA Online, February 2007.
Retrieved from "http://en.wikipedia.org/wiki/Euler%27s_identity"
Personal tools