List of moments of inertia

From Wikipedia, the free encyclopedia

The following is a list of moments of inertia. Mass moments of inertia have units of dimension mass × length². It is the rotational analogue to mass. It should not be confused with the second moment of area (area moment of inertia), which is used in bending calculations. The following moments of inertia assume constant density throughout the object.

NOTE: The axis of rotation is taken to be through the center of mass, unless otherwise specified.

Description	Moment(s) of inertia	Comment
Thin cylindrical shell with open ends, of radius r and mass m	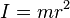$I = m r^2 \,\!$	This expression assumes the shell thickness is negligible. It is a special case of the next object for r₁=r₂.
Thick-walled cylindrical tube with open ends, of inner radius r₁, outer radius r₂, length h and mass m	$I_z = \frac{1}{2} m\left({r_1}^2 + {r_2}^2\right)$ ^[1] $I_x = I_y = \frac{1}{12} m\left[3\left({r_1}^2 + {r_2}^2\right)+h^2\right]$ or when defining the normalized thickness t_n = t/r and letting r = r₂, then $I_z = mr^2\left(1-t_n+\frac{1}{2}t_n^2\right)$	With a density of ρ and the same geometry $I_z = \frac{1}{2} \pi\rho h\left({r_2}^4 - {r_1}^4\right)$
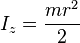Solid cylinder of radius r, height h and mass m	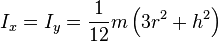$I_z = \frac{m r^2}{2}\,\!$ $I_x = I_y = \frac{1}{12} m\left(3r^2+h^2\right)$	This is a special case of the previous object for r₁=0. Keep in mind that m in this case is greater than in the previous one.
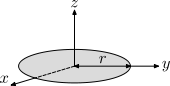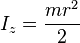Thin, solid disk of radius r and mass m	$I_z = \frac{m r^2}{2}\,\!$ $I_x = I_y = \frac{m r^2}{4}\,\!$	This is a special case of the previous object for h=0.
Thin circular hoop of radius r and mass m	$I_z = m r^2\!$ $I_x = I_y = \frac{m r^2}{2}\,\!$	This is a special case of torus object for b=0.
Solid sphere of radius r and mass m	$I = \frac{2 m r^2}{5}\,\!$	A sphere can be taken to be made up of a stack of infinitesimal thin, solid discs, where the radius differs from 0 to r.
Hollow sphere of radius r and mass m	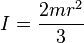$I = \frac{2 m r^2}{3}\,\!$	Similar to the solid sphere, only this time considering a stack of infinitesimal thin, circular hoops.
Oblate Spheroid of major a, minor b and mass m	$I = \frac{2 m b^2}{3}\,\!$	—
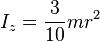Right circular cone with radius r, height h and mass m	$I_z = \frac{3}{10}mr^2 \,\!$ $I_x = I_y = \frac{3}{5}m\left(\frac{r^2}{4}+h^2\right) \,\!$	—
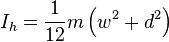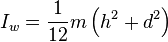Solid cuboid of height h, width w, and depth d, and mass m	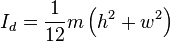$I_h = \frac{1}{12} m\left(w^2+d^2\right)$ $I_w = \frac{1}{12} m\left(h^2+d^2\right)$ $I_d = \frac{1}{12} m\left(h^2+w^2\right)$	For a similarly oriented cube with sides of length $s$ , $I_{CM} = \frac{m s^2}{6}\,\!$ .
Thin rectangular plane of height h and of width w and mass m	$I_c = \frac {m(h^2 + w^2)}{12}$	—
Thin rectangular plane of height h and of width w and mass m (Axis of rotation at the end of the plate)	$I_e = \frac {m(h^2)}{3}+\frac {m(w^2)}{12}$	—
Rod of length L and mass m	$I_{\mathrm{center}} = \frac{m L^2}{12} \,\!$	This expression assumes that the rod is an infinitely thin (but rigid) wire. This is a special case of the previous object for w=L and h=d=0.
Rod of length L and mass m (Axis of rotation at the end of the rod)	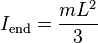$I_{\mathrm{end}} = \frac{m L^2}{3} \,\!$	This expression assumes that the rod is an infinitely thin (but rigid) wire.
Torus of tube radius a, cross-sectional radius b and mass m.	About a diameter: 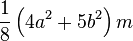$\frac{1}{8}\left(4a^2 + 5b^2\right)m$ About the vertical axis: 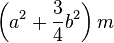$\left(a^2 + \frac{3}{4}b^2\right)m$	—
Plane polygon with vertices 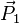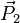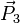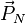$\vec{P}_{1}$ , $\vec{P}_{2}$ , $\vec{P}_{3}$ , ..., $\vec{P}_{N}$ and mass $m$ uniformly distributed on its interior, rotating about an axis perpendicular to the plane and passing through the origin.	$I=\frac{m}{6}\frac{\sum_{n=1}^{N}\|\|\vec{P}_{n+1}\times\vec{P}_{n}\|\|(\vec{P}^{2}_{n+1}+\vec{P}_{n+1}\cdot\vec{P}_{n}+\vec{P}_{n}^{2})}{\sum_{n=1}^{N}\|\|\vec{P}_{n+1}\times\vec{P}_{n}\|\|}$	—