American wire gauge

From Wikipedia, the free encyclopedia

Jump to: navigation, search
A standard wire gauge.

American wire gauge (AWG), also known as the Brown & Sharpe wire gauge, is a standardized wire gauge system used since 1857 predominantly in the United States for the diameters of round, solid, nonferrous, electrically conducting wire.[1] The cross-sectional area of each gauge is an important factor for determining its current-carrying capacity.

Contents

The steel industry uses different wire gauges (for example, W&M Wire Gauge or US Steel Wire Gauge, or Music Wire Gauge). The table below does not apply to steel wire.

Increasing gauge numbers give decreasing wire diameters, which is similar to many other non-metric gauging systems. This gauge system originated in the number of drawing operations used to produce a given gauge of wire. Very fine wire (for example, 30 gauge) requires more passes through the drawing dies than does 0 gauge wire. Manufacturers of wire formerly had proprietary wire gauge systems; the development of standardized wire gauges rationalized selection of wire for a particular purpose.

The AWG tables are for a single, solid, round conductor. The AWG of a stranded wire is determined by the total cross-sectional area of the conductor, which determines its current-carrying capacity and electrical resistance. Because there are also small gaps between the strands, a stranded wire will always have a slightly larger overall diameter than a solid wire with the same AWG.

The AWG size is one of the essential specifications that are printed on data cables. For instance, an AWG of 24 is common for network cables such as a Category 5 UTP, and an AWG of 26 is the norm for Serial ATA cables.

AWG is also commonly used to specify body piercing jewelry sizes, especially smaller sizes.[2]

[edit] Formula

By definition, No. 36 AWG is 0.0050 inches in diameter, and No. 0000 is 0.4600 inches in diameter. The ratio of these diameters is 92, and there are 40 gauge sizes from No. 36 to No. 0000, or 39 steps. Using this common ratio, wire gauge sizes vary geometrically according to the following formula: The diameter of a No. n AWG wire is

d_n = 0.005~\mathrm{inch} \times 92 ^ \frac{36-n}{39} = 0.127~\mathrm{mm} \times 92 ^ \frac{36-n}{39}

the gauge can be calculated from the diameter using

n = -39\log_{92} \left( \frac{d_{n}}{0.005~\mathrm{inch}} \right)+36 = -39\log_{92} \left( \frac{d_{n}}{0.127~\mathrm{mm}} \right)+36 [3]

and the cross-section area is

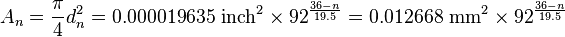A_n = \frac{\pi}{4} d_n^2 = 0.000019635~\mathrm{inch}^2 \times 92 ^ \frac{36-n}{19.5} = 0.012668~\mathrm{mm}^2 \times 92 ^ \frac{36-n}{19.5}.

Sizes with multiple zeros are successively larger than No. 0 and can be denoted using "number of zeros/0", for example 4/0 for 0000. For an m/0 AWG wire, use n = −(m−1) = 1−m in the above formulas. For instance, for No. 0000 or 4/0, use n = −3.

The ASTM B 258-02 standard defines the ratio between successive sizes to be the 39th root of 92, or approximately 1.1229322.[4] ASTM B 258-02 also dictates that wire diameters should be tabulated with no more than 4 significant figures, with a resolution of no more than 0.0001 inches (0.1 mils) for wires larger than No. 44 AWG, and 0.00001 inches (0.01 mils) for wires No. 45 AWG and smaller.

[edit] Rules of Thumb

The sixth power of this ratio is very close to 2,[5] which leads to the following rules of thumb:

  • When the diameter of a wire is doubled, the AWG will decrease by 6. (e.g. No. 2 AWG is about twice the diameter of No. 8 AWG.)
  • When the cross-sectional area of a wire is doubled, the AWG will decrease by 3. (e.g. Two No. 14 AWG wires have about the same cross-sectional area as a single No. 11 AWG wire.)

Additionally, a decrease of ten gauge numbers, for example from No. 10 to 1/0, multiplies the area and weight by approximately 10 and reduces the resistance by approximately 10.

[edit] Table of AWG wire sizes

The table below shows various data including both the resistance of the various wire gauges and the allowable current (ampacity) based on plastic insulation. The diameter information in the table applies to solid wires. Stranded wires are calculated by calculating the equivalent cross sectional copper area. The table below assumes DC, or AC frequencies equal to or less than 60 Hz, and does not take skin effect into account. Turns of wire is on a best-case scenario when winding tightly packed coils with no insulation.

AWG Diameter Turns of wire Area Copper
resistance[6]		 NEC copper wire
ampacity with 60/75/
90°C insulation (A)[7]		 Approximate
stranded metric
equivalents
(inch) (mm) (per inch) (per cm) (kcmil) (mm²) (Ω/km) (Ω/kFT)
0000 (4/0) 0.4600 11.684 2.17 0.856 212 107 0.1608 0.04901 195 / 230 / 260
000 (3/0) 0.4096 10.404 2.44 0.961 168 85.0 0.2028 0.06180 165 / 200 / 225
00 (2/0) 0.3648 9.266 2.74 1.08 133 67.4 0.2557 0.07793 145 / 175 / 195
0 (1/0) 0.3249 8.252 3.08 1.21 106 53.5 0.3224 0.09827 125 / 150 / 170
1 0.2893 7.348 3.46 1.36 83.7 42.4 0.4066 0.1239 110 / 130 / 150
2 0.2576 6.544 3.88 1.53 66.4 33.6 0.5127 0.1563 95 / 115 / 130
3 0.2294 5.827 4.36 1.72 52.6 26.7 0.6465 0.1970 85 / 100 / 110 196/0.4
4 0.2043 5.189 4.89 1.93 41.7 21.2 0.8152 0.2485 70 / 85 / 95
5 0.1819 4.621 5.50 2.16 33.1 16.8 1.028 0.3133 126/0.4
6 0.1620 4.115 6.17 2.43 26.3 13.3 1.296 0.3951 55 / 65 / 75
7 0.1443 3.665 6.93 2.73 20.8 10.5 1.634 0.4982 80/0.4
8 0.1285 3.264 7.78 3.06 16.5 8.37 2.061 0.6282 40 / 50 / 55
9 0.1144 2.906 8.74 3.44 13.1 6.63 2.599 0.7921 84/0.3
10 0.1019 2.588 9.81 3.86 10.4 5.26 3.277 0.9989 30 / 35 / 40
11 0.0907 2.305 11.0 4.34 8.23 4.17 4.132 1.260 56/0.3
12 0.0808 2.053 12.4 4.87 6.53 3.31 5.211 1.588 25 / 25 / 30 (20)
13 0.0720 1.828 13.9 5.47 5.18 2.62 6.571 2.003 50/0.25
14 0.0641 1.628 15.6 6.14 4.11 2.08 8.286 2.525 20 / 20 / 25 (15)
15 0.0571 1.450 17.5 6.90 3.26 1.65 10.45 3.184 30/0.25
16 0.0508 1.291 19.7 7.75 2.58 1.31 13.17 4.016 — / — / 18 (10)
17 0.0453 1.150 22.1 8.70 2.05 1.04 16.61 5.064 32/0.2
18 0.0403 1.024 24.8 9.77 1.62 0.823 20.95 6.385 — / — / 14 (7) 24/0.2
19 0.0359 0.912 27.9 11.0 1.29 0.653 26.42 8.051
20 0.0320 0.812 31.3 12.3 1.02 0.518 33.31 10.15 16/0.2
21 0.0285 0.723 35.1 13.8 0.810 0.410 42.00 12.80
22 0.0253 0.644 39.5 15.5 0.642 0.326 52.96 16.14 7/0.25
23 0.0226 0.573 44.3 17.4 0.509 0.258 66.79 20.36
24 0.0201 0.511 49.7 19.6 0.404 0.205 84.22 25.67 1/0.5, 7/0.2, 30/0.1
25 0.0179 0.455 55.9 22.0 0.320 0.162 106.2 32.37
26 0.0159 0.405 62.7 24.7 0.254 0.129 133.9 40.81 7/0.15
27 0.0142 0.361 70.4 27.7 0.202 0.102 168.9 51.47
28 0.0126 0.321 79.1 31.1 0.160 0.0810 212.9 64.90
29 0.0113 0.286 88.8 35.0 0.127 0.0642 268.5 81.84
30 0.0100 0.255 99.7 39.3 0.101 0.0509 338.6 103.2 1/0.25, 7/0.1
31 0.00893 0.227 112 44.1 0.0797 0.0404 426.9 130.1
32 0.00795 0.202 126 49.5 0.0632 0.0320 538.3 164.1 1/0.2, 7/0.08
33 0.00708 0.180 141 55.6 0.0501 0.0254 678.8 206.9
34 0.00630 0.160 159 62.4 0.0398 0.0201 856.0 260.9
35 0.00561 0.143 178 70.1 0.0315 0.0160 1079 329.0
36 0.00500 0.127 200 78.7 0.0250 0.0127 1361 414.8
37 0.00445 0.113 225 88.4 0.0198 0.0100 1716 523.1
38 0.00397 0.101 252 99.3 0.0157 0.00797 2164 659.6
39 0.00353 0.0897 283 111 0.0125 0.00632 2729 831.8
40 0.00314 0.0799 318 125 0.00989 0.00501 3441 1049

The "Approximate stranded metric equivalents" column lists commonly available cables in the format "number of strands / diameter of individual strand (mm)" which is the common nomenclature describing cable construction within an overall cross-sectional area. Some common cables are midway between two AWG sizes. Cables sold in Europe are normally labeled according to the combined cross section of all strands in mm², which can be compared directly with the Area column.

In the North American electrical industry, conductors larger than 4/0 AWG are generally identified by the area in thousands of circular mils (kcmil), where 1 kcmil = 0.5067 mm². A circular mil is the area of a wire one mil in diameter. One million circular mils is the area of a cylinder with 1000 mil = 1 inch diameter. An older abbreviation for one thousand circular mils is MCM.

Outside North America, wire sizes for electrical purposes are usually given as the cross sectional area in square millimeters. International standard manufacturing sizes for conductors in electrical cables are defined in IEC 60228.

Note that the area in mm² may differ somewhat from the numbers given in the table, depending on number of strands etc.

[edit] Pronunciation

AWG is colloquially referred to as gauge and the zeros in large wire sizes are referred to as aught (pronounced /ɔːt/). Wire sized 1 AWG is referred to as "one gauge"; similarly, smaller diameters are pronounced "x gauge", where x is the positive integer AWG number. Larger wire (#0 and up) is referred to as "one aught", "two aught" etc, depending on how many zeros are in the AWG rating.[8]

[edit] See also

[edit] References

  1. ^ ASTM Standard B 258-02, Standard specification for standard nominal diameters and cross-sectional areas of AWG sizes of solid round wires used as electrical conductors, ASTM International, 2002
  2. ^ Body Piercing Jewelry Size Reference — SteelNavel.com
  3. ^ The logarithm to the base 92 can be computed using any other logarithm, such as common or natural logarithm, using log92x = (log x)/(log 92).
  4. ^ ASTM Standard B 258-02, page 4
  5. ^ The result is roughly 2.0050, or one-quarter of one percent higher than 2
  6. ^ Figure for solid copper wire at 68 °F, computed based on 100% IACS conductivity of 58.0 MS/m, which agrees with multiple sources: High-purity oxygen-free copper can achieve up to 101.5% IACS conductivity; e.g. the Kanthal conductive alloys data sheet lists slightly lower resistances than this table.
  7. ^ NFPA 70 National Electrical Code 2008 Edition. Table 310.16 page 70-148, Allowable ampacities of insulated conductors rated 0 throrugh 2000 volts, 60°C through 90°C, not more than three current-carrying conductors in raceway, cable, or earth (directly buried) based on ambient temperature of 30°C. Extracts from NFPA 70 do not represent the full position of NFPA and the original complete Code must be consulted.
  8. ^ Glossary of Power Terms | Event Solutions

[edit] Further reading

  • Donald G. Fink and H. Wayne Beaty, Standard Handbook for Electrical Engineers, Eleventh Edition,McGraw-Hill, New York, 1978, ISBN 0-07-020974-X, page 4-18 and table 4-11.

[edit] External links

Retrieved from "http://en.wikipedia.org/wiki/American_wire_gauge"
Personal tools