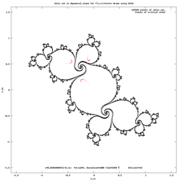
Julia set
From Wikipedia, the free encyclopedia
| This article contains too much jargon and may need simplification or further explanation. Please discuss this issue on the talk page, and/or remove or explain jargon terms used in the article. Editing help is available. (March 2009) |
In complex dynamics, the Julia set  [1] of a holomorphic function f informally consists of those points whose long-time behavior under repeated iteration of f can change drastically under arbitrarily small perturbations (bifurcation locus).
[1] of a holomorphic function f informally consists of those points whose long-time behavior under repeated iteration of f can change drastically under arbitrarily small perturbations (bifurcation locus).
The Fatou set  of f is the complement of the Julia set: that is, the set of points which exhibit 'stable' behavior.
of f is the complement of the Julia set: that is, the set of points which exhibit 'stable' behavior.
Thus on  , the behavior of
, the behavior of 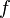 is 'regular', while on
is 'regular', while on  , it is 'chaotic'.
, it is 'chaotic'.
These sets are named after the French mathematicians Gaston Julia,[2] and Pierre Fatou[3] who initiated the theory of complex dynamics in the early 20th century.
Contents |
[edit] Formal definition
Let
be a holomorphic map of a Riemann surface X to itself. Assume that X is either the Riemann sphere, the complex plane, or the once-punctured complex plane, as the other cases do not give rise to interesting dynamics. (Such maps are completely classified.)
Consider f as a discrete dynamical system on the phase space X, and consider the behavior of the iterates fn of f (that is, the n-fold compositions of f with itself).
The Fatou set of f consists of all points  such that the family of iterates
such that the family of iterates
forms a normal family in the sense of Montel when restricted to some open neighborhood of z.
The Julia set of f is the complement of the Fatou set in X.
[edit] Equivalent descriptions of the Julia set
- J(f) is the smallest closed set containing at least three points which is completely invariant under f.
- J(f) is the closure of the set of repelling periodic points.
- For all but at most two points
 , the Julia set is the set of limit points of the full backwards orbit
, the Julia set is the set of limit points of the full backwards orbit  . (This suggests a simple algorithm for plotting Julia sets, see below.)
. (This suggests a simple algorithm for plotting Julia sets, see below.) - If f is an entire function - in particular, when f is a polynomial, then J(f) is the boundary of the set of points which converge to infinity under iteration.
- If f is a polynomial, then J(f) is the boundary of the filled Julia set; that is, those points whose orbits under f remain bounded.
[edit] Properties of the Julia set and Fatou set
The Julia set and the Fatou set of f are both completely invariant under holomorphic function f, i.e.
- f − 1(J(f)) = f(J(f)) = J(f)
and
- f − 1(F(f)) = f(F(f)) = F(f).[4]
[edit] Rational maps

There has been extensive research on the Fatou set and Julia set of iterated rational functions, known as rational maps. For example, it is known that the Fatou set of a rational map has either 0,1,2 or infinitely many components.[5] Each component of the Fatou set of a rational map can be classified into one of four different classes.[6]
[edit] Quadratic polynomials
A very popular complex dynamical system is given by the family of quadratic polynomials, a special case of rational maps. The quadratic polynomials can be expressed as
where c is a complex parameter.
|
Filled Julia set for fc, c=1−φ where φ is the golden ratio |
|||

The parameter plane of quadratic polynomials - that is, the plane of possible c-values - gives rise to the famous Mandelbrot set. Indeed, the Mandelbrot set is defined as the set of all c such that J(fc) is connected. For parameters outside the Mandelbrot set, the Julia set is a Cantor set: in this case it is sometimes referred to as Fatou dust.
In many cases, the Julia set of c looks like the Mandelbrot set in sufficiently small neighborhoods of c. This is true, in particular, for so-called 'Misiurewicz' parameters, i.e. parameters c for which the critical point is pre-periodic. For instance:
- At c = i, the shorter, front toe of the forefoot, the Julia set looks like a branched lightning bolt.
- At c = − 2, the tip of the long spiky tail, the Julia set is a straight line segment.
In other words the Julia sets J(fc) are locally similar around Misiurewicz points.[7]
[edit] Generalizations
The definition of Julia and Fatou sets easily carries over to the case of certain maps whose image contains their domain; most notably transcendental meromorphic functions and Epstein's 'finite-type maps'.
Julia sets are also commonly defined in the study of dynamics in several complex variables.
[edit] Plotting the Julia set
[edit] Using backwards (inverse) iteration (IIM)
As mentioned above, the Julia set can be found as the set of limit points of the set of pre-images of (essentially) any given point. So we can try to plot the Julia set of a given function as follows. Start with any point z we know to be in the Julia set, such as a repelling periodic point, and compute all pre-images of z under some high iterate fn of f.
Unfortunately, as the number of iterated pre-images grows exponentially, this is not computationally feasible. However, we can adjust this method, in a similar way as the "random game" method for iterated function systems. That is, in each step, we choose at random one of the inverse images of 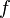 .
.
For example, for the quadratic polynomial  , the backwards iteration is described by
, the backwards iteration is described by
At each step, one of the two square roots is selected at random.
Note that certain parts of the Julia set are quite hard to reach with the reverse Julia algorithm. For this reason, one must modify IIM/J ( it is called MIIM/J) or use other methods usually produce better images.
[edit] Using DEM/J
[edit] See also
| Wikimedia Commons has media related to: Julia set |
| Wikibooks has a book on the topic of |
- Mandelbrot set
- Limit set
- Stable and unstable sets
- No wandering domain theorem
- Fatou components
- Chaos theory
[edit] Notes
- ^ Note that in other areas of mathematics the notation
 can also represent the Jacobian matrix of a real valued mapping
can also represent the Jacobian matrix of a real valued mapping 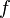 between smooth manifolds.
between smooth manifolds. - ^ Gaston Julia (1918) "Mémoire sur l'iteration des fonctions rationnelles," Journal de Mathématiques Pures et Appliquées, vol. 8, pages 47-245.
- ^ Pierre Fatou (1917) "Sur les substitutions rationnelles," Comptes Rendus de l'Académie des Sciences de Paris, vol. 164, pages 806-808 and vol. 165, pages 992-995.
- ^ Beardon, Iteration of Rational Functions, Theorem 3.2.4
- ^ Beardon, Iteration of Rational Functions, Theorem 5.6.2
- ^ Beardon, Theorem 7.1.1
- ^ Lei.pdf Tan Lei, "Similarity between the Mandelbrot set and Julia Sets", Communications in Mathematical Physics 134 (1990), pp. 587-617.
[edit] References
- Lennart Carleson and Theodore W. Gamelin, Complex Dynamics, Springer 1993
- Adrien Douady and John H. Hubbard, "Etude dynamique des polynômes complexes", Prépublications mathémathiques d'Orsay 2/4 (1984 / 1985)
- John W. Milnor, Dynamics in One Complex Variable (Third Edition), Annals of Mathematics Studies 160, Princeton University Press 2006 (First appeared in 1990 as a Stony Brook IMS Preprint, available as arXiV:math.DS/9201272.)
- Alexander Bogomolny, "Mandelbrot Set and Indexing of Julia Sets" at cut-the-knot.
- Evgeny Demidov, "The Mandelbrot and Julia sets Anatomy" (2003)
- Alan F. Beardon, Iteration of Rational Functions, Springer 1991, ISBN 0-387-95151-2
[edit] External links
- Eric W. Weisstein, Julia Set at MathWorld.
- Julia Set Fractal (2D), Paul Burke
- The Julia Set in Four Dimensions
- Julia Sets, Jamie Sawyer
- Julia Jewels: An Exploration of Julia Sets, Michael McGoodwin
- Crop circle Julia Set, Lucy Pringle
- Interactive Julia Set Applet
- Julia and Mandelbrot Set Explorer, David E. Joyce
- A simple program to generate Julia sets (Windows, 370 kb)
- A collection of applets one of which can render Julia sets via Iterated Function Systems.