Odds
From Wikipedia, the free encyclopedia
In probability theory and statistics the odds in favour of an event or a proposition are the quantity 
, where p is the probability of the event or proposition. The odds against the same event are 
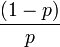. For example, if you chose a random day of the week (7 days), then the odds that you would choose a Sunday would be:
, but not 
.
The odds against you choosing Sunday are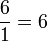 
, meaning that it's 6 times more likely that you don't choose Sunday. These 'odds' are actually relative probabilities. Generally, 'odds' are not quoted to the general public in this format because of the natural confusion with the chance of an event occurring being expressed fractionally as a probability. Thus, the probability of choosing Sunday at random from the days of the week is 'one-seventh' (1/7). A bookmaker may (for his own purposes) use 'odds' of 'one-sixth', the overwhelming everyday use by most people is odds of the form 6 to 1, 6-1, or 6/1 (all read as 'six-to-one') where the first figure represents the number of ways of failing to achieve the outcome and the second figure is the number of ways of achieving a favourable outcome: thus these are "odds against". In other words, an event with m to n "odds against" would have probability n/(m + n), while an event with m to n "odds on" would have probability m/(m + n). However, even in probability theory, odds may play a more natural or a more convenient role than probabilities. This is in particular the case in problems of sequential decision making as for instance in problems of how to stop (online) on a last specific event which is solved by the Odds algorithm.
In some games of chance, this is also the most convenient way for a person to understand how much winnings will be paid if the selection is successful: the person will be paid 'six' of whatever stake unit was bet for each 'one' of the stake unit wagered. For example, a £10 winning bet at 6/1 will win '6 × £10 = £60' with the original £10 stake also being returned.
Taking an event with a 1 in 5 probability of occurring (i.e. a probability of 1/5, 0.2 or 20%), then the odds are 0.2 / (1 − 0.2) = 0.2 / 0.8 = 0.25. This figure (0.25) represents the stake necessary for a person to win one unit on a successful wager. This may be scaled up by any convenient factor to give whole number values. E.g. If a stake of 0.25 wins 1 unit, then scaling by a factor of four means a stake of 1 wins 4 units. If you bet 1 at these odds and the event occurred, you would receive back 4 plus your original 1 stake. This would be presented in fractional odds of 4 to 1 against (written as 4-1, 4:1, or 4/1), in decimal odds as 5.0 to include the returned stake, in craps payout as 5 for 1, and in moneyline odds as +400 representing the gain from a 100 stake.
By contrast, for an event with a 4 in 5 probability of occurring (i.e. a probability of 4/5, 0.8 or 80%), then the odds are 0.8 / (1 − 0.8) = 4. If you bet 4 at these odds and the event occurred, you would receive back 1 plus your original 4 stake. This would be presented in fractional odds of 4 to 1 on (written as 1/4 or 1-4), in decimal odds as 1.25 to include the returned stake, in craps as 5 for 4, and in moneyline odds as −400 representing the stake necessary to gain 100.
[edit] Gambling odds versus probabilities
In gambling, the odds on display do not represent the true chances that the event will occur, but are the amounts that the bookmaker will pay out on winning bets. In formulating his odds to display the bookmaker will have included a profit margin which effectively means that the payout to a successful punter is less than that represented by the true chance of the event occurring. This profit is known as the 'over-round' on the 'book' (the 'book' relates to the old-fashioned ledger that wagers were recorded in and thus gives us the term 'bookmaker') and relates to the sum of the 'odds' in the following way:
In a 3-horse race, for example, the true chances of each of the horses winning based on their relative abilities may be 50%, 40% and 10%. These are the relative probabilities of the horses winning and are simply the bookmaker's 'odds' multiplied by 100 for convenience. The total of these three percentages is 100, thus representing a fair 'book'. The true odds of winning for each of the three horses is evens, 6-4 and 9-1 respectively. In order to generate a profit on the wagers accepted by the bookmaker he may decide to increase the values to 60%, 50% and 20% for the three horses, representing odds of 4-6, Evens and 4-1. These values now total 130, meaning that the book has an overround of 30 (130 − 100). This value of 30 represents the amount of profit for the bookmaker if he accepts bets in the correct proportions on each of the horses. The art of bookmaking is that he will take in, for example, $130 in wagers and only pay $100 back (including stakes) no matter which horse wins.
Profiting in gambling involves predicting the relationship of the true probabilities to the payout odds. If you can consistently make bets where the odds of paying out are better (pay out more) than the true odds of the event, then over time (in theory) you will come out ahead. Sports information services are often used by professional and semi-professional sports bettors to help achieve this goal.
The odds or amounts the bookmaker will pay are determined by the amounts bet on each of the respective possible events. They reflect the balance of wagers on either side of the event, and include the deduction of a bookmaker’s brokerage fee (“vig” or vigorish).
[edit] Even odds
The terms 'even odds', 'even money' or simply 'Evens' imply that the payout will be 'one-for-one' or 'double-your-money'. Assuming there is no bookmaker’s fee or built-in profit margin, this means that the actual probability of winning is 50%. The term “better than even odds” looks at it from the perspective of a gambler rather than a statistician. If the odds are Evens (1-1), and you bet 10, you would win 10. If the gamble was paying 4-1 and the event occurred, you would make a profit of 40. So, it is better than Evens from the gambler’s perspective because it pays out more than one-for-one. If an event is more positively favored to occur than a 50-50 chance then the odds will be worse than Evens, and the bookmaker will pay out less than one-for-one.
In popular parlance surrounding uncertain events, the expression "better than even" usually implies a better than (greater than) 50% chance of the event occurring, which is exactly the opposite of the meaning of the expression when used in a gaming context.
The odds are a ratio of probabilities; an odds ratio is a ratio of odds, that is, a ratio of ratios of probabilities. Odds-ratios are often used in analysis of clinical trials. While they have useful mathematical properties, they can produce counter-intuitive results: in the example above an event with an 80% probability of occurring is four times more likely to happen than an event with a 20% probability, but the odds are actually 16 times higher on the less likely event (4-1 against) than on the more likely one (1-4, or 4-1 on).
The logarithm of the odds is the logit of the probability.
