Resonance

From Wikipedia, the free encyclopedia

Jump to: navigation, search
 This article needs additional citations for verification. Please help improve this article by adding reliable references (ideally, using inline citations). Unsourced material may be challenged and removed. (October 2007)
This article is about resonance in physics. For other uses, see Resonance (disambiguation).
"Resonant" redirects here. For the phonetic term, see Sonorant.
Increase of amplitude as damping decreases and frequency approaches resonance frequency.[1]

In physics, resonance is the tendency of a system to oscillate at its maximum amplitude, associated with specific frequencies known as the system's resonance frequencies (or resonant frequencies). At these frequencies, even small periodic driving forces can produce large amplitude vibrations, because the system stores vibrational energy. When damping is small, the resonance frequency is approximately equal to the natural frequency of the system, which is the frequency of free vibrations. Resonant phenomena occur with all types of vibrations or waves: there is mechanical resonance, acoustic resonance, electromagnetic resonance, NMR, ESR and resonance of quantum wave functions. Resonant systems can be used to generate vibrations of a specific frequency, or pick out specific frequencies from a complex vibration containing many frequencies.

Resonance was discovered by Galileo Galilei with his investigations of pendulums beginning in 1602.

Contents

[edit] Examples

One familiar example is a playground swing, which acts as a pendulum. Pushing a person in a swing in time with the natural interval of the swing (its resonance frequency) will make the swing go higher and higher (maximum amplitude), while attempts to push the swing at a faster or slower tempo will result in smaller arcs. This is because the energy the swing absorbs is maximized when the pushes are 'in phase' with the swing's oscillations, while some of the swing's energy is actually extracted by the opposing force of the pushes when they are not.

Resonance occurs widely in nature, and is exploited in many man-made devices. It is the mechanism by which virtually all sinusoidal waves and vibrations are generated. Many sounds we hear, such as when hard objects of metal, glass, or wood are struck, are caused by brief resonant vibrations in the object. Light and other short wavelength electromagnetic radiation is produced by resonance on an atomic scale, such as electrons in atoms. Other examples are:

[edit] Theory

For a linear oscillator with a resonance frequency Ω, the intensity of oscillations I when the system is driven with a driving frequency ω is given by:

I(\omega) \propto \frac{\frac{\Gamma}{2}}{(\omega - \Omega)^2 + \left( \frac{\Gamma}{2} \right)^2 }.

The intensity is defined as the square of the amplitude of the oscillations. This is a Lorentzian function, and this response is found in many physical situations involving resonant systems. Γ is a parameter dependent on the damping of the oscillator, and is known as the linewidth of the resonance. Heavily damped oscillators tend to have broad linewidths, and respond to a wider range of driving frequencies around the resonance frequency. The linewidth is inversely proportional to the Q factor, which is a measure of the sharpness of the resonance.

[edit] Resonators

A physical system can have as many resonance frequencies as it has degrees of freedom; each degree of freedom can vibrate as a harmonic oscillator. Systems with one degree of freedom, such as a mass on a spring, pendulums, balance wheels, and LC tuned circuits have one resonance frequency. Systems with two degrees of freedom, such as coupled pendulums and resonant transformers can have two resonance frequencies. As the number of coupled harmonic oscillators grows, the time it takes to transfer energy from one to the next becomes significant. The vibrations in them begin to travel through the coupled harmonic oscillators in waves, from one oscillator to the next.

Extended objects that experience resonance due to vibrations inside them are called resonators, such as organ pipes, vibrating strings, quartz crystals, microwave cavities, and laser rods. Since these can be viewed as being made of millions of coupled moving parts (such as atoms), they can have millions of resonance frequencies. The vibrations inside them travel as waves, at an approximately constant velocity, bouncing back and forth between the sides of the resonator. If the distance between the sides is d\,, the length of a round trip is 2d\,. In order to cause resonance, the phase of a sinusoidal wave after a round trip has to be equal to the initial phase, so the waves will reinforce. 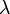So the condition for resonance in a resonator is that the round trip distance, 2d\,, be equal to an integral number of wavelengths \lambda\, of the wave:

2d = N\lambda,\qquad\qquad N \in \{1,2,3...\}

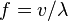If the velocity of a wave is v\,, the frequency is f = v / \lambda\, so the resonance frequencies are:

f = \frac{Nv}{2d}\qquad\qquad N \in \{1,2,3...\}

So the resonance frequencies of resonators, called normal modes, are equally spaced multiples of a lowest frequency called the fundamental frequency. The multiples are often called overtones. There may be several such series of resonance frequencies, corresponding to different modes of vibration.

[edit] Failure of the original Tacoma Narrows Bridge

Main article: Tacoma Narrows Bridge (1940)

The dramatically visible, rhythmic twisting that resulted in the 1940 collapse of "Galloping Gertie," the original Tacoma Narrows Bridge, has sometimes been characterized in physics textbooks as a classical example of resonance; however, this description is misleading. The catastrophic vibrations that destroyed the bridge were not due to simple mechanical resonance, but to a more complicated oscillation between the bridge and the winds passing through it — a phenomenon known as aeroelastic flutter. Robert H. Scanlan, father of the field of bridge aerodynamics, wrote an article about this misunderstanding.[2]

For more details on this topic, see mechanical resonance.

[edit] Resonances in quantum mechanics

In quantum mechanics and quantum field theory resonances may appear in similar circumstances to classical physics. However, they can also be thought of as unstable particles, with the formula above still valid if the Γ is the decay rate and Ω replaced by the particle's mass M. In that case, the formula just comes from the particle's propagator, with its mass replaced by the complex number M + iΓ. The formula is further related to the particle's decay rate by the optical theorem.

This section requires expansion.

[edit] String resonance in music instruments

Main article: String resonance (music)

String resonance occurs on string instruments. Strings or parts of strings may resonate at their fundamental or overtone frequencies when other strings are sounded. For example, an A string at 440 Hz will cause an E string at 330 Hz to resonate, because they share an overtone of 1320 Hz (the third overtone of A and fourth overtone of E).

[edit] Resonance causing vibration on the International Space Station

The rocket engines for the International Space Station are controlled by autopilot. Ordinarily the uploaded parameters for controlling the engine control system for the Zvezda module will cause the rocket engines to boost the International Space Station to a higher orbit. The rocket engines are hinge-mounted, and ordinarily the operation is not noticed by the crew. But on January 14, 2009, the uploaded parameters caused the autopilot to swing the rocket engines in larger and larger oscillations, at a 2 second frequency. These oscillations were captured on video, and lasted for 142 seconds.[3]

[edit] See also

Electronics portal
Physics portal

[edit] References

  1. ^ Ogata, Katsuhiko. System Dynamics 4th Edition. University of Minnesota. 2004. (p. 617)
  2. ^ K. Billah and R. Scanlan (1991), Resonance, Tacoma Narrows Bridge Failure, and Undergraduate Physics Textbooks, American Journal of Physics, 59(2), 118--124 (PDF)
  3. ^ Continued Shaking on space station rattles NASA.

[edit] External links

Retrieved from "http://en.wikipedia.org/wiki/Resonance"
Personal tools