Introduction to quantum mechanics
From Wikipedia, the free encyclopedia
Quantum mechanics (QM, or quantum theory) is a branch of physics dealing with the behavior of matter and energy on the minute scale of atoms and subatomic particles. Quantum mechanics is fundamental to our understanding of all of the fundamental forces of nature except gravity.
Quantum mechanics is the foundation of several branches of physics, including electromagnetism, particle physics, condensed matter physics, and even parts of cosmology. Quantum mechanics is also essential to the theory of chemical bonding (and hence all of chemistry), structural biology, and technologies such as electronics, information technology, and nanotechnology. A century of experiments and of work in applied science has proved quantum mechanics successful and practical.
Quantum mechanics began in the early 20th century, with the pathbreaking work of Max Planck and Niels Bohr. Max Born coined the term "quantum mechanics" in 1924. The wider physics community soon accepted quantum mechanics because of its highly accurate empirical predictions, especially in systems where Newtonian mechanics fails. A major early success of quantum mechanics was its explanation of wave-particle duality, namely of how subatomic particles have wave-like properties and waves have particle-like properties. Quantum mechanics also applies to a wider range of situations than general relativity does, e.g., to systems whose scale is atomic or smaller, and to those having very low or very high energies or subjected to the lowest temperatures.
Contents |
[edit] Overview
[edit] The unexpected
In the late 19th century, classical physics appeared to some as nearly complete, but this perception was challenged by empirical findings that such physics could not explain. Physical theories that worked very well for situations on the human scale in space and time failed to explain situations that were very small, very massive, or that moved at very high velocities. A view of the universe that had, by and large, distilled commonplace observations was challenged by observations and theories that predicted correctly where classical physics had given impossible results. But the emerging picture was of a universe that stubbornly refused to behave in a way that agreed with human common sense.
On the large end of the scale, relativity theory said that time does not pass at the same rate for all observers, that matter can convert to energy and vice-versa, that two objects each moving at more than half the speed of light cannot approach each other at a speed exceeding the speed of light, that time progresses more slowly near massive objects, etc. Things just did not work in a way that experience with scales, rulers, and clocks here on earth had led humans to expect.
In the small, the wonders were even more abundant. A photon or electron has neither a location nor a traceable trajectory between the point where it is emitted and the point where it is detected. The points where such a particle may be detected are not where one would expect them to be based on everyday experience. With a small probability, the detection point can even be on the other side of a solid barrier. Probability turns out to be a highly salient factor in all interactions on this scale. The trajectory of any atomic scale object is "squishy" in the sense that any measurement that makes an object's position more precise reduces the precision with which we can observe its velocity, and vice-versa.
In the era of classical physics, Newton and his followers believed that light consisted of a stream of particles, and others believed that light consisted of waves propagating through some medium. Rather than finding an experiment that would prove one or the other side correct, physicists discovered that an experiment designed to show light's frequency or other wave "characteristics" will manifest light's "wave nature," while an experiment designed to show its momentum or other particle "characteristics" will reveal light's "particle nature." Furthermore, objects as large as atoms, and even some molecules, have revealed a "wave nature" when observed in the appropriate way.
The most eminent of physicists have warned that if an explanation of quantum physics "makes sense", then that explanation is very likely to be flawed.[citation needed] In 1927 Niels Bohr wrote: "Anyone who is not shocked by quantum theory does not understand it."
[edit] How the unexpected came to light
The foundations of quantum mechanics (QM) arguably began with the earliest work on the properties of light, in the 17th century, and the discovery of the properties of electricity and magnetism, early in the 19th century. In 1690, Christiaan Huygens employed a wave theory to explain the reflection and refraction of light.[1] Isaac Newton believed that light consisted of infinitesimally small particles which he called "corpuscles." In 1827, Thomas Young and Augustin Fresnel conducted experiments on light interference that found results that were inconsistent with a corpuscular theory of light. All theoretical and empirical results through the late 19th century seemed inconsistent with Newton's corpuscular theory of light.
Later experiments identified phenomena, such as the photoelectric effect, that were consistent only with a packet or "quantum" model of light. When light strikes an electrical conductor, electrons seem to move away from their original positions. These observations can only be explained by assuming that light delivers energy in definite packets. In a photoelectric device such as the light meter in a camera, light hitting the metallic detector causes electrons to move. Increasing the intensity of light having a single frequency will cause more electrons to move. But making the electrons move faster requires increasing the frequency of the light. Thus the intensity of light controls the electric current flowing through the circuit, while its frequency controls the circuit's voltage. These observations contradicted the wave model of light derived from the study of sound waves and ocean waves, where the intensity of the initial impulse sufficed to predict the energy of the resulting wave. In the case of light, energy was solely a function of frequency, a fact which begged for an explanation. It was also necessary to reconcile experiments showing the particle nature of light with other experiments revealing its wave nature.
In 1874, George Johnstone Stoney was the first to propose that a physical quantity, namely electric charge, could not vary by less than some irreducible amount. Thus electric charge was the first physical quantity to be theoretically quantized. In 1873, James Clerk Maxwell showed theoretically that an oscillating electrical circuit should produce electromagnetic waves. Given Maxwell's equations, it was possible to calculate the speed of electromagnetic radiation purely from electrical and magnetic measurements, and the calculated value corresponded very closely to the measured speed of light.[2] In 1888, Heinrich Hertz made an electrical device that produced radiation whose frequency was lower than that of visible light, radiation we would now call microwaves.[3] Early researchers differed in how they explained the fundamental nature of what we now call electromagnetic radiation, some maintaining that it is composed of particles, while others asserted that it is a wave phenomenon. In classical physics, these ideas are mutually exclusive.

QM formally began with Max Planck's landmark 1900 paper on black body radiation,[4] marking the first appearance of the quantum hypothesis. Planck's work made clear that neither the wave nor the particle model can explain electromagnetic radiation. In 1905, Albert Einstein extended Planck's theory to explain the photoelectric effect.[5] In 1913, Niels Bohr set out his theory of the atom, one incorporating Planck's quantum hypothesis in an essential way.[6] This and other early 20th century work makes up the "old quantum theory."
In 1924, Louis de Broglie posited the matter-wave hypothesis. This hypothesis proved to be a turning point, and quickly led to a more sophisticated and complete variant of QM.[7] Important contributors in the mid-1920s to what came to be called the "new quantum mechanics" or "new physics" were Max Born,[8] Paul Dirac,[9] Werner Heisenberg,[10] Wolfgang Pauli,[11] and Erwin Schrödinger.[12] In the late 1940s and early 1950s, Julian Schwinger, Sin-Itiro Tomonaga, Richard Feynman, and Freeman Dyson discovered quantum electrodynamics, which significantly advanced our understanding of the quantum theory of electromagnetism and the electron. Later, Murray Gell-Mann developed a related theory of the strong force, called quantum chromodynamics.
[edit] Spectroscopy and onward
When white light passes through a prism, or the bevelled edge of a mirror or a tapered pane of glass, or through drops of rain to form a rainbow, the white light is decomposed into a spectrum. This spectrum reveals that white light is composed of light of all colours and hence of all frequencies.
When a sample composed of a pure chemical element emits light by heating or other agency, the spectrum of the emitted light, called the emission spectrum, is peculiar to that element and the temperature to which it is heated. Unlike the spectrum of white light, an emission spectrum is not a wide band composed of all the colours from indigo to red, but instead consists of narrow bands, each of a single colour and separated from other bands by darkness. Such a display is called a line spectrum. An emission spectrum can also contain lines outside the range of visible light, detectable only by special photographic film or electronic devices.
It was hypothesized that an atom emits electromagnetic radiation the way a violin string "radiates" sound – not only with a fundamental frequency (in which the entire string moves the same way at once), but also with higher harmonics (formed when the string divides itself into halves and other divisions that vibrate in coordination with each other as when one half of the string is going one way as the other half of the string is going the opposite way). A mathematical description of the line spectrum of an element proved elusive until 1885, when Johann Jakob Balmer proposed the following simple formula to describe the line spectrum of atomic hydrogen:
where λ is wavelength, R is the Rydberg constant, and n is an integer > 2. This formula can be generalized to apply to atoms other than hydrogen, a fact that will not detain us, except to note that this is the reason why the denominator in the first fraction is expressed as a square.
The next development was Pieter Zeeman's discovery of the Zeeman effect, whose physical explanation was worked out by Hendrik Antoon Lorentz. Lorentz hypothesized that the line spectrum of hydrogen resulted from vibrating electrons. It is possible to obtain information about what goes on within the atom, because moving electrons create a magnetic field. Hence an electron can be influenced by an external magnetic field, similar to the way that an iron magnet will attract or repel another magnet.
The Zeeman effect could be interpreted to mean that the line spectrum results from electrons vibrating in their orbits, but classical physics could not explain why an electron does not spiral into the nucleus, nor why electron orbits have the properties required to produce observed line spectra, describable by Balmer's formula. More pointedly, why do electrons behave in such a way that emission spectra are not continuous but instead are line spectra?
[edit] Old quantum theory
Quantum mechanics grew out of the spectroscopic study of electromagnetic waves. The most familiar form of such waves is visible light. Other forms can be more energetic than light, such as ultraviolet light, x-rays, and gamma rays. Forms that are less energetic (and thus have longer wavelengths) than light include infrared waves, microwaves, and radio waves. All electromagnetic waves propagate in a vacuum at the speed of light. Henceforth, "particle" always refers to elementary or subatomic particles.
[edit] Planck's constant
Classical physics predicted that a black-body radiator would emit an infinite amount of energy. Not only was this prediction absurd, but the observed emission spectrum of a black-body rose from zero at one end, peaked at a frequency related to the temperature of the radiator, and then declined to zero. In 1900, Max Planck developed an empirical equation that could account for the observed emission spectra of black bodies, but could not reconcile that equation with classical physics. He concluded that, contrary to what was generally believed at the time, classical physics does not apply on the microscopic scale.
Planck's theory allowed all possible frequencies and wavelengths, while restricting the energy delivered. "In classical physics,... the energy of a given oscillator depends merely on its amplitude, and this amplitude is subject to no restriction."[13] Planck's theory also concluded that the energy emitted by a radiator is strictly proportional to its frequency, and the higher the frequency, the greater the energy. To reach this conclusion, he postulated that a radiating macroscopic body consisted of an enormous number of elementary oscillators, each vibrating at some frequency between zero and infinity. (It was later confirmed that these elementary oscillators are atoms or molecules.) Planck further assumed that the energy E of any one oscillator was proportional to some integral multiple of its frequency f. That is,
where n =1, 2, 3,... h is a fundamental physical constant first proposed by Planck and now named Planck's constant in his honor.[14] h is exceedingly small, about 6.6260693 × 10-34 joule-seconds.
If h and the frequency of a photon are known, then this equation can be employed to calculate the photon's energy. For instance, if a beam of light has a frequency of 540 × 1012 hertz, then the energy of each photon making up the beam would be h × (540 × 1012 hertz). Hence the photons in the beam of light have an energy of about 3.58 × 10-19 joules, equivalent to about 2.23 eV.
When the energy of a wave is described in this manner, the wave appears to carry its energy in little packets. Planck's name for these packets of energy was quanta. Planck's discovery remade electromagnetic waves into particles. Quantum mechanics began with the discovery that electromagnetic energy is quantized, that is, is emitted in quanta, whose size is related to the frequency of the associated electromagnetic wave. In the case of visible light, energy is related to color, because color is strictly determined by frequency. However, the reader should keep in mind that these descriptions in terms of packet, wave, and particle import macroscopic concepts into the quantum world, where their value is no more than suggestive.
In early research on light, there were two competing ways to describe light: as waves propagated through empty space, or as small particles traveling in straight lines. Because Planck showed that the energy of light waves is quantized, the particle analogy became favored, as it helped us understand how light delivers energy in multiples of certain set values, called quanta of energy. Nevertheless, the wave analogy remained indispensable for helping to understand other light phenomena, such as diffraction.
In 1905, Albert Einstein used Planck's constant to explain the photoelectric effect by postulating that the energy in a beam of light occurs in packets he called light quanta, and that later came to be called photons.[15] According to Einstein's account, a single photon of a given frequency delivers an invariant amount of energy. In other words, individual photons can deliver more or less energy, but only depending on their frequencies. Although this description that built on Planck's theory sounds like Newton's corpuscular account, Einstein's photon was still said to have a frequency, with the energy of the photon being proportional to its frequency. Once again, the particle account of light had been "compromised."[16]
Both the idea of a wave and the idea of a particle are models derived from our everyday experience. We cannot see individual photons, and can only investigate their properties indirectly. Take, for example, the rainbow of colours we see reflected from a puddle of water when a thin film of oil rests on its surface. We can explain that phenomenon by modelling light as waves.[17] Other phenomena, such as the working of the photoelectric meters in our cameras, may be explained by thinking in terms of particles of light colliding with the detection screen inside the meter. In both cases, we take concepts from our everyday experience and apply them to a world we will never see or otherwise experience directly.
Neither wave nor particle is an entirely satisfactory explanation. In general, any model can only approximate that which it models. A model is useful only within the range of conditions where it makes accurate predictions. Newtonian physics remains a good predictor of most everyday (macroscopic) phenomena. To remind us that both "wave" and "particle" are concepts imported from our macro world to explain atomic-scale phenomena, physicists such as Banesh Hoffmann have used the term "wavicle" to refer to whatever it is that is "really there." In the following discussion, "wave" and "particle" may both be used depending on which aspect of quantum mechanical phenomena is under discussion.
[edit] Reduced Planck's (or Dirac's) constant
Planck's constant was, originally, the factor of proportionality linking the energy that a light wave carries, and its frequency. A step in the development of this concept appeared in Bohr's work. Bohr was using a "planetary" or particle model of the electron, and at first could not understand why the factor 2π kept turning up in his experimentally derived algebraic formulas.
Later, de Broglie hypothesized that electrons, like photons, have frequencies. Moreover, the frequency of an electron must conform to the conditions for a standing wave that can exist in a certain orbit. That is to say, the beginning of one cycle of a wave at some point on the circumference of a circle (since that is what an orbit is) must coincide with the end of some cycle. There can be no gap, no length along the circumference that is not participating in the vibration, and there can be no overlap of cycles. So the circumference of the orbit, C, must equal the wavelength, λ, of the electron multiplied by some positive integer (n = 1, 2, 3...). Knowing the circumference one can calculate wavelengths that fit that orbit, and knowing the radius, r, of the orbit, one can calculate its circumference. In algebra:
Solving for λ:
This formula is expressed in terms of the radius r, when what is relevant to determining allowed frequencies and wavelengths is the circumference C. Hence 2π recurs in QM because it is the factor of proportionality linking the radius of any circle to its circumference.[18]
In 1925, when Werner Heisenberg was developing his wave formulation of full quantum theory, calculations involving Fourier series were fundamental, and the factor 2π is ubiquitous in the algebra of Fourier series. Adopting the "reduced" version of Planck's constant (h/2π) eliminated most appearances of 2π from wave analysis algebra. A few years later, the reduced Planck's constant appeared naturally in Dirac's equation, and for this reason it was named "Dirac's constant." We now say more about this constant, even though the theories for which it was more convenient than Planck's constant have yet to be discussed.
As noted above, the energy of any wave is given by its frequency multiplied by Planck's constant. A wave is made up of peaks and troughs. A complete cycle for a wave is the time required for a wave to return to some chosen initial position. For example, starting from a peak, a wave is complete when it has its next peak. A cycle is mathematically related to a circle, and both have 360 degrees. A degree is a unit of measure for the amount of turn needed to produce an arc equal to 1/360 of the circumference. A point on the circumference of a circle traces out a sine curve as the circle rotates. (For a demonstration, see Rotation Applet.)
Now let the length of an arc of a circle equal the length of a radius of the circle. Connect the endpoints of this arc to the center of the circle. Then the angle between these two radii equals 1 radian. Hence both circles and wave cycles consist of 2π radians. Since one cycle equals 2π radians, when h is divided by 2π, the two "2 π" factors will cancel out, leaving just a variable measured in radians to contend with. So dividing h by 2π yields a constant that when multiplied by the frequency of a wave, gives the energy of the wave in joules per radian. The reduced Planck's constant, called "h bar," is written as:
-
 .
.
The reduced Planck's constant allows computing the energy of a wave in units per radian instead of in units per cycle. The constants h and ħ merely serve to convert frequency units into energy units.
The reduced Planck's constant appears more often than h in the algebra of QM for many reasons, one of which is that angular velocity or angular frequency is ordinarily measured in radians per second, so using ħ eliminates converting radians into degrees or vice-versa. Also, when QM equations are written in terms of ħ, the frequent 2π factors in numerator and denominator often cancel. However, in other cases, as in the orbits of the Bohr atom, h/2π arises naturally from the algebra of orbital angular momentum.
The numerical value of h depends on the choice of units in which energy and wavelength are measured. If energy is measured in electron volts (eV, a common practice in particle physics) and wavelength is measured in ångströms (10-10m), then the energy of a photon is approximately EeV = 12400/λångström. This form is easily remembered and avoids the small values of SI units.[19]
[edit] Bohr atom
In 1897, a research team headed by J J Thompson discovered and named the electron, the carrier of negative charge. By means of the gold foil experiment, physicists discovered that matter is mostly empty space.[20] Once that was clear, it was hypothesized that negatively charged electrons orbit a positively charged nucleus, so that all atoms resemble a miniature solar system. But that simple analogy predicted that electrons would take only about one hundredth of a microsecond[21] to crash into the nucleus. Hence the great question of early 20th century physics was: "How do electrons normally remain in stable orbits around the nucleus?"
In 1913, Niels Bohr solved this substantial problem by applying the notion of discrete (non-continuous) quanta to electron orbits. This solution became known as the Bohr model of the atom. Bohr basically theorized that electrons can only inhabit certain orbits around the atom. These orbits could be derived by looking at the spectral lines produced by pure elements.[22]
Bohr explained the orbits that electrons can take by relating the angular momentum of electrons in each "permitted" orbit to the value of h, Planck's constant. He held that an electron in the lowest orbital has a discrete angular momentum equal to h/2π. Each orbit after the initial orbit must provide for an electron's angular momentum being an integer multiple of that lowest value. He depicted electrons in atoms as being analogous to planets in solar orbit. However, he took h to be a fundamental quantity that introduces special requirements at this subatomic level, and explains the spacing of the electron orbits.
Bohr considered one revolution in orbit to be equivalent to one cycle in an oscillator (as in Planck's initial measurements of h) which is in turn similar to one cycle in a wave. The number of revolutions per second is (or defines) what we call the frequency of that electron or that orbit. Specifying that the frequency of each orbit must be an integer multiple of h would not only limit the possible orbits but would also fix their size.
Bohr generalized Balmer's formula for hydrogen by replacing the denominator in the term 1/4 with an explicit squared variable:
 m=1,2,3,4,5,..., and n > m,
m=1,2,3,4,5,..., and n > m,
where λ is the wavelength of the light, RH is the Rydberg constant for hydrogen, and n and m are integers referring to the orbits between which electrons can transit. This generalization predicted many more line spectra than had been previously detected, and experimental confirmation of this prediction followed.
It follows almost immediately that if λ is quantized as the formula above indicates, then the momentum of any photon must be quantized. The frequency of light, ν, at a given wavelength λ is given by the relationship  which can be rearranged as
which can be rearranged as  Because
Because  we can rewrite the preceding equation as
we can rewrite the preceding equation as 
Let E = pc, where p denotes momentum. Solving for p yields  Substituting p for E/c above yields
Substituting p for E/c above yields  which can be rearranged to obtain
which can be rearranged to obtain 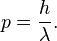
Beginning with line spectra, physicists were able to deduce empirically the rules according to which the orbits of electrons are determined, and to discover something essential about the momenta involved — namely that they are quantized.[23]
Bohr next realized how the angular momentum of an electron in its orbit, L, is quantized, i.e., he determined that there is some constant value K such that when it is multiplied by h, it will yield the angular momentum that pertains to the lowest orbital. Multiplying Kh by successive integers gives the angular momentum for other possible orbitals. Bohr later determined that K = 1/2π. (A detailed argument can be found here.)
Bohr proposed that when an electron changed orbits, it did not move in a continuous trajectory from one orbit around the nucleus to another. Instead, it suddenly disappeared from its original orbit and reappeared in another orbit. Each distance at which an electron can orbit is a function of a quantized amount of energy. The closer to the nucleus an electron orbits, the less energy it takes to remain in that orbit. Electrons that absorb a photon gain a quantum of energy, so they jump to an orbit that is farther from the nucleus, while electrons that emit a photon lose a quantum of energy and so jump to an orbital closer to the nucleus. Electrons cannot gain or lose a fractional quantum of energy, hence they cannot be found at some fraction of the distance between allowed orbits. The values of n are natural numbers, and correspond to allowed orbitals, with the innermost orbit designated n = 1, the next being n = 2, and so on.
Bohr's theory represented electrons as orbiting the nucleus of an atom, much as planets orbit around he sun. However, we now envision electrons circulating around the nuclei of atoms in a way that is strikingly different from Bohr's atom, and what we see in the world of our everyday experience. Instead of orbits, electrons inhabit "orbitals," which describe probability distributions rather than discrete positions of electrons orbiting around atomic nuclei.
Bohr's model of the atom was essentially two-dimensional: an electron orbiting in a plane around its nuclear "sun." Modern theory [24] describes a three-dimensional arrangement of electronic shells and orbitals around atomic nuclei. The orbitals are spherical (s-type) or lobular (p, d and f-types) in shape. It is the underlying structure and symmetry of atomic orbitals, and the way that electrons fill them, that determines the structure and strength of chemical bonds between atoms. Thus, the bizarre quantum nature of the atomic and sub-atomic world is expressed in the macroscopic world we are more familiar with.
[edit] Wave-particle duality
Niels Bohr showed that neither the wave analogy nor the particle analogy, taken individually, fully describe the empirical properties of light. All forms of electromagnetic radiation were found to behave in certain experiments as though they were particles, and in other experiments as though they were waves. With these facts in mind, Bohr enunciated the principle of complementarity, which pairs concepts such as wave and particle, or position and momentum.
In 1924, Louis de Broglie explored the mathematical consequences of Bohr's findings and discovered the theory of wave-particle duality, which states that subatomic particles too have simultaneous wave and particle properties. De Broglie expanded the Bohr model of the atom by showing that an electron in orbit around a nucleus could be thought of as having wave-like properties. In particular, an electron will be observed only in situations that permit a standing wave around a nucleus. An example of a standing wave is a string fixed at both ends and made to vibrate (as in a string instrument). Hence a standing wave must have zero amplitude at each fixed end. The waves created by a stringed instrument also appear to oscillate in place, moving from crest to trough in an up-and-down motion. A standing wave requires that the vibrating entity have a length equal to an integer multiple of the length of the vibrating entity. In a vibrating medium that traces out a simple closed curve, the wave must be a continuous formation of crests and troughs all around the curve. Since electron orbitals are simple closed curves, each electron must be its own standing wave, occupying a unique orbital.
[edit] Development of modern quantum mechanics
[edit] Full quantum mechanical theory
The complete theory of quantum mechanics first appeared 1925, in the work of Werner Heisenberg (1901-1976), who in 1932 won the Nobel Prize in Physics.
Following his mentor Bohr, Heisenberg devised a quantum theory of electron orbitals. Because electrons could not be observed in their orbits, Heisenberg devised a mathematical description of quantum mechanics built on what could be observed, namely the characteristic line spectra of atoms. Heisenberg modelled the electron orbital using the idea of a charged ball on a spring -- an oscillator -- whose motion is anharmonic (not quite regular). (For a picture of the behavior of a charged ball on a spring see: Vibrating Charges.) Heisenberg first explained this kind of observed motion in terms of the laws of classical mechanics (whose applicability to macro phenomena no one disputed), then adding quantum restrictions, discrete (non-continuous) properties. Doing so caused gaps to appear between the predicted orbitals, so that the mathematical description he formulated represented only the electron orbitals predicted on the basis of atomic spectra.
In approaching the problem that Bohr gave him to solve, Heisenberg took the strategic stance of not dealing with unobservable quantities, and formulating equations using only quantities that could be observed. That strategy led him to begin with the actual experimental evidence at hand. By this time, there was good data on:
- The frequencies (and hence energies) emitted or absorbed by electron transitions from one of the Bohr stationary orbits, revealed by the positions of the lines in line spectra;
- The "transition amplitude" or likelihood of transition from one orbit to another, revealed by the the strength of the lines in line spectra, etc.
Starting from classical formulas characterizing those phenomena, Heisenberg devised analogous formulas that took account of quantum conditions. Formulas that followed from decisions made up to this point explained known empirical findings well, but also predicted new but unexpected findings. In his paper introducing quantum mechanics, he cautioned:
"A significant difficulty arises, however, if we consider two quantities x(t), y(t), and ask after their product... Whereas in classical x(t)y(t) is always equal to y(t)x(t), this is not necessarily the case in quantum theory."[25]
When the predicted values are exhibited in matrix form and multiplications are performed, the nature of the difficulty appears in a form familiar to mathematicians. More significantly, empirical studies validate the theoretical results and reveal that the difference between x(t)y(t) and y(t)x(t) has a value related to Planck's constant.
Schema for a table of transition frequencies (produced when electrons change orbitals):
| Electron States | S1 | S2 | S3 | S4 | S5 | .... | |
|---|---|---|---|---|---|---|---|
| S1 | f1→1 | f2→1 | f3→1 | f4→1 | f5→1 | ..... | |
| S2 | f1→2 | f2→2 | f3→2 | f4→2 | f5→2 | ..... | |
| S3 | f1→3 | f2→3 | f3→3 | f4→3 | f5→3 | ..... | |
| S4 | f1→4 | f2→4 | f3→4 | f4→4 | f5→4 | ..... | |
| S5 | f1→5 | f2→5 | f3→5 | f4→5 | f5→5 | ..... | |
| S..... | ..... | ..... | ..... | ..... | ..... | ..... |
Schema for a related table showing the transition amplitudes:
| Electron States | S1 | S2 | S3 | S4 | S5 | .... | |
|---|---|---|---|---|---|---|---|
| S1 | a1→1 | a2→1 | a3→1 | a4→1 | a5→1 | ..... | |
| S2 | a1→2 | a2→2 | a3→2 | a4→2 | a5→2 | ..... | |
| S3 | a1→3 | a2→3 | a3→3 | a4→3 | a5→3 | ..... | |
| S4 | a1→4 | a2→4 | a3→4 | a4→4 | a5→4 | ..... | |
| S5 | a1→5 | a2→5 | a3→5 | a4→5 | a5→5 | ..... | |
| S..... | ..... | ..... | ..... | ..... | ..... | ..... |
Heisenberg then developed mathematical ways of summarizing the information in tables such as those above.[26] Empirically filling in the values for tables involving quantum quantities is not a simple procedure, since any measurement made on a single system gives a value of one characteristic of the system, but has the potential of changing the values of other characteristics. So large numbers of identical copies of the system in question must be prepared, and a single measure made on each system. Multiple experiments to determine the same characteristics are made, and the data from all the experiments are averaged. Even then, precise measurements of all simultaneous characteristics of the system are unattainable, because of quantum uncertainty. A precise determination of the value of one characteristic necessarily gives rise to an uncertainty in the value of its correlate. "Certain pairs of observables simply cannot be simultaneously measured to an arbitrarily high level of precision." If simultaneous measurements are made of correlated characteristics (such as position and momentum) in multiple identical systems, the product of the measurements will inevitably differ by an amount greater than or equal to  ."[27]
."[27]
In 1925, Heisenberg published a paper titled "Quantum-mechanical re-interpretation of kinematic and mechanical relations" relating his discoveries.[28] This paper was the end of the old quantum theory, and the start of QM. Heisenberg's paper gave few details that might aid readers in determining how he actually obtained his results for the one-dimensional models he used to form his hypothesis that proved so useful. In his paper, Heisenberg proposed to "discard all hope of observing hitherto unobservable quantities, such as the position and period of the electron," and instead restricted himself to observable quantities. He needed mathematical rules for predicting the relations actually observed in nature, and the rules he produced worked differently depending on the sequence in which they were applied. About this Aitchison has written: "It quickly became clear that the 'non-commutativity' (in general) of kinematical quantities in quantum theory was the really essential new technical idea in the paper."[29]
The special type of multiplication that Heisenberg's formulae required was matrix multiplication, with matrices being rectangular arrays of numbers, as in a table. In the everyday multiplication of numbers, the order does not matter, so that xy = yx. Mathematicians say that the multiplication of numbers commutes. However, matrix multiplication does not commute. If A and B are two matrices, the product AB generally does not equal BA. (This was valuable for QM, because measuring velocity before position yields a different result than if position is measured first.) The matrix formulation turned out to be a convenient way of organizing information and making clear the exact sequence in which calculations were made. It gave a nice symbolic form to certain unexpected empirical findings.
Heisenberg approached QM from the historical perspective that treated an electron as an oscillating charged particle. Bohr's use of this analogy had already allowed him to explain why the radii of the orbits of electrons could only take on certain values. It followed from this interpretation of the available experimental results and Heisenberg's subsequent quantum theory, that an electron could not be between two "permitted" orbits. Therefore, Heisenberg described electrons as "jumping" from orbit to orbit. The idea that an electron might now be in one place and an instant later be in some other place without having traveled between the two points was one of the earliest indications of the "spookiness" of quantum phenomena. Although the scale is vastly smaller, "jumping" from orbit to orbit is as strange and unexpected as someone walking out of a London building onto a Los Angeles street. The phenomenon of quantum tunneling is another example of how electrons seem able to move in the "spooky" way that Heisenberg ascribed to their behavior within atoms.
Variables having a period of 2π, like a cycle in a wave, are called Fourier series variables; position and momentum are cases in point. Heisenberg's matrix mechanics attributed to the electron "wave" the particle-like properties of position and momentum. The product of measured position and momentum is intensity. Heisenberg found, however, that the product of position and momentum, taken in that order, differed from the product of momentum and position, again taken in that order. The difference between the two calculated intensities was h/2π. Two years would pass before Heisenberg understood the reason for this difference, but for the time being he satisfied himself that the math worked, and exactly described the quantum behavior of the electron.
Heisenberg's matrix mechanics was the first complete formulation of the laws and properties of QM, and it fully described the known behavior of the electron. It was later extended to all subatomic particles. Very soon after matrix mechanics was introduced, Schrödinger independently set out a quantum wave theory that appeared to have no resemblance whatsoever to matrix mechanics. However, it was computationally easier and avoided some of the odd-sounding ideas of matrix mechanics, such as the electron making a "quantum leap" from one orbital to another. But Schrödinger soon showed that the two theories made the same predictions in essentially all situations. Finally, Dirac devised yet another formulation of QM, one in which noncommutativity played a central role, and proved the formulations of Heisenberg and of Schrödinger to be special cases of his own.
[edit] Schrödinger wave equation
In 1925, building on De Broglie's theoretical model of particles as waves, Erwin Schrödinger analyzed how an electron would behave if it were assumed to be a wave surrounding a nucleus. Rather than explaining the atom by an analogy to satellites orbiting a planet, he treated electrons as waves with each electron having a unique wavefunction. Such wavefunctions were named "Schrödinger's equation" in his honor. Schrödinger's equation describes a wavefunction by three properties (Wolfgang Pauli later added a fourth: spin):
- An "orbital" designation, indicating whether the particle wave is one that is closer to the nucleus with less energy or one that is farther from the nucleus with more energy;
- The "shape" of the orbital, spherical or otherwise;
- The "inclination" of the orbital, determining the magnetic moment of the orbital around the z-axis.
The collective name for these three properties is the "wavefunction of the electron," describing the quantum state of the electron. The quantum state of an electron refers to its collective properties, which describe what can be said about the electron at a point in time. The quantum state of the electron is described by its wavefunction, denoted by the Greek letter ψ ("psi," pronounced "sigh").
The three properties of Schrödinger's equation describing the wavefunction of the electron (and thus its quantum state) are each called quantum numbers. The first property describing the orbital is the principal quantum number, numbered according to Bohr's model, in which n denotes the energy of each orbital.
The next quantum number, the azimuthal quantum number, denoted l (lower case L), describes the shape of the orbital. The shape is a consequence of the angular momentum of the orbital. The rate of change of the angular momentum of any system is equal to the resultant external torque acting on that system. In other words, angular momentum represents the resistance of a spinning object to speeding up or slowing down under the influence of external force. The azimuthal quantum number "l" represents the orbital angular momentum of an electron around its nucleus. However, the shape of each orbital has its own letter as well. The first shape is denoted by the letter s (for "spherical"). The next shape is denoted by the letter p and has the form of a dumbbell. The other orbitals have more complicated shapes (see Atomic Orbitals), and are denoted by the letters d, f, and g. The entry carbon atom describes the orbitals of carbon.
The third quantum number in Schrödinger's equation describes the magnetic moment of the electron. This number is denoted by either m or m with a subscript l, because the magnetic moment depends on the second quantum number l.
In May 1926, Schrödinger proved that Heisenberg's matrix mechanics and his own wave mechanics made the same predictions about the properties and behaviour of the electron; mathematically, the two theories were identical. Yet both men disagreed on the physical interpretations of their respective theories. Heisenberg saw no problem in the existence of discontinuous quantum jumps, while Schrödinger hoped that a theory based on continuous wave-like properties could avoid what he called (in the words of Wilhelm Wien[30]), "this nonsense about quantum jumps."
[edit] Uncertainty principle
In 1927, Heisenberg employed his matrix mechanics to discover a fact that had practical consequences for matter and energy on the atomic scale. He found that a certain formula yielded a discrepancy of h/4π between the measured position of a particle, and its measured momentum. The smaller the error in the measured position of a particle, the greater the error in its measured momentum, with h/4π being the lower limit of the uncertainty involved. This conclusion came to be called Heisenberg's Uncertainty Principle.
Quantum mechanics strongly limits the precision with which the properties of moving subatomic particles can be measured. An observer can precisely measure one of position or momentum, but not both. In the limit, measuring either variable with complete precision would entail a complete absence of precision in the measurement of the other.
Heisenberg, in a voice recording of an early lecture on the uncertainty principle, said of the Bohr model of the atom:
"You can say, well, this orbit is really not a complete orbit. Actually at every moment the electron has only an inaccurate position and an inaccurate velocity and between these two inaccuracies there is this uncertainty relation. And only by this idea it was possible to say what such an orbit was." [31]
One consequence of the uncertainty principle was that the electron could no longer be viewed as having an exact location in its orbital, at some instant of time. Rather, one had to evaluate every possible location for the electron. Calculating points of probable location for the electron, given some orbital, gave rise to a depiction of the hydrogen atom orbital as a spherically shaped "cloud of points," with the cloud having a maximum density at a certain distance from the nucleus, and growing less dense at greater and lesser distances. Mathematicians refer to such a cloud of points as a probability distribution. The Bohr atom number n for each orbital became known as an n-sphere, pictured as a probability cloud surrounding the nucleus.
If the position of an electron defies measurement, then it cannot be described as having any particular location. All we can do is to assign a probability to the electron's being in some region of its orbital. In other words, quantum mechanics can only give probabilities for the occurrence of of possible outcomes. Heisenberg went so far as to argue that the path of a moving particle comes into existence only when we observe the particle! He was speaking of the particle itself, not its orbital. However paradoxical and counterintuitive his assertion may seem, quantum mechanics still yields the probability distribution for an electron's location within a given orbital.
While Heisenberg's matrices allow an electron to have an infinite number of possible locations, this does not mean that the electron can be anywhere in the universe. Rather there are several reasons why the electron must occupy a single localized probability distribution. Bohr's theory described an electron by its energy level, and matrix mechanics concurred. Therefore, an electron in a certain n-sphere has to be within a certain range of distances from the nucleus, a range determined by its energy. This fact restricts its location. The number of places an electron can be is also called "the number of cells in its phase space." The Uncertainty Principle set a lower limit to how finely one can divide a classical phase space, so that the number of locations in an orbital that an electron can possibly occupy becomes finite. An electron's location in an atom is defined by its orbital, but an orbital stops at the nucleus, and short of where the next spherical orbital begins.
Classical physics since Newton had shown that if the position and motions of stars and planets were known, then where they will be in the future can be predicted. The uncertainty principle implies that this is not possible for subatomic particles. One cannot know the precise position and momentum of a particle at a given instant, and all that can be known about the position and motion of a particle at some future moment is a probability distribution.
These consequences of the uncertainty principle only arise at the subatomic level and follow from wave-particle duality. Despite having these counterintuitive implications, quantum mechanics with its uncertainty principle has resulted in major technological advances, including computers, fluorescent lights, and medical imaging devices.
[edit] Wavefunction collapse
Schrödinger's wave equation with its unique wavefunction for a single electron is also spread out in a probability distribution like Heisenberg's quantized particle-like electron. This is because a wave is naturally a widespread disturbance and not a point particle. Therefore, Schrödinger's wave equation makes the same predictions as the uncertainty principle, because uncertainty of location is built into the definition of a widespread disturbance like a wave. Uncertainty only needed to be defined from Heisenberg's matrix mechanics because the treatment was from the particle-like aspects of the electron. Schrödinger's wave equation shows that the electron is in the probability cloud at all times, in its probability distribution as a wave that is spread out.
Max Born discovered in 1928 that when Schrödinger's wavefunction is squared (to yield psi-squared), the result is a probability distribution for the electron's location.[32] Therefore to the extent that the position of an electron can be measured exactly instead of as a probability distribution, the electron momentarily ceases to have wave-like properties. Without wave-like properties, none of Schrödinger's assumptions about the wave-like character of the electron make sense. The measurement of the position of the particle nullifies the simple wave-like properties, so that the one-body form of Schrödinger's equation fails. Because the electron can no longer be described by its separate wavefunction when measured, due to its wave length becoming much shorter and to its becoming entangled with the particles of the measuring apparatus, the wavefunction is said to collapse.
[edit] Eigenstates and eigenvalues
Because of the uncertainty principle, statements about both the position and momentum of particles can only assign a probability that the position or momentum will have some numerical value. The uncertainty principle also says that eliminating uncertainty about either position or momentum maximizes uncertainty about the second. A probability distribution assigns probabilities to all possible values of position and momentum. Schrödinger's wave equation gives wavefunction solutions, which are probabilities of where the electron might be, just as Heisenberg's probability distribution does.
In the everyday world, it is natural and intuitive to think of everything being in its own eigenstate. Everything appears to have a definite position, a definite momentum, a definite measured value, a definite time of occurrence. However, the uncertainty principle says that it is impossible to measure the exact value for the momentum of some particle like an electron, given that its position has been determined at a given instant. Likewise, it is impossible to determine the exact location of that particle once its momentum has been measured at a particular instant.
Therefore it became necessary to formulate clearly the difference between the state of something that is uncertain in the way just described, such as an electron in a probability cloud, and the state of something having a definite value. When an object can definitely be "pinned-down" in some respect, it is said to possess an eigenstate. As stated above, when the wavefunction collapses because the position of an electron has been determined, the electron's state becomes an "eigenstate of position," meaning that its position has a known value, an eigenvalue of the eigenstate of position.
The word "eigenstate" is derived from the German/Dutch word "eigen," meaning "inherent" or "characteristic." An eigenstate is the measured state of some object possessing quantifiable characteristics such as position, momentum, etc. The state being measured and described must be observable (i.e. something such as position or momentum that can be experimentally measured either directly or indirectly), and must have a definite value, called an eigenvalue. ("Eigenvalue" also refers to a mathematical property of square matrices, a usage pioneered by the mathematician David Hilbert in 1904. Square matrices are crucial to matrix mechanics.)
[edit] The Pauli exclusion principle
The Pauli Exclusion Principle states that each fermion in an atom must have a unique quantum description. A very consequential corollary is that in any atom, two electrons cannot occupy the same quantum state.
Wolfgang Pauli proposed the following concise statement of Pauli's principle:
"There cannot exist an atom in such a quantum state that two electrons within have the same set of quantum numbers."[33]
Wolfgang Pauli developed the Exclusion Principle from what he called a "two-valued quantum degree of freedom" to account for the observation of a doublet, meaning a pair of lines differing by a small amount (e.g., on the order of 0.15Å), in the spectrum of atomic hydrogen. The observation meant that there was more energy in the electron orbital from magnetic moments than had previously been described.
In early 1925, Uhlenbeck and Goudsmit proposed that electrons rotate about an axis in the same way that the earth rotates on its axis. They proposed to call this property spin. Spin would account for the missing magnetic moment, and allow two electrons in the same orbital to occupy distinct quantum states if they "spun" in opposite directions, thus satisfying the Exclusion Principle. A new quantum number was then needed, one to represent the momentum embodied in the rotation of each electron.
We have established that an electron has four quantum numbers:
- n, the principal quantum number;
- l, the azimuthal quantum number;
- ml, the magnetic quantum number;
- ms, the spin quantum number.
Pauling wrote, by way of example:
- "In the case of a helium atom with two electrons in the 1 s orbital, the Pauli Exclusion Principle requires that the two electrons differ in the value of one quantum number. Their values of n, l, and ml are the same; moreover, they have the same spin quantum number, s = 1/2. Accordingly they must differ in the value of ms, which can have the value of +½ for one electron and -½ for the other."[33]
[edit] Dirac wave equation
In 1928, Paul Dirac extended the Pauli equation, which described spinning electrons, to account for special relativity. The result was a theory that dealt properly with events, such as the speed at which an electron orbits the nucleus, occurring at a substantial fraction of the speed of light. By using the simplest electromagnetic interaction, Dirac was able to predict the value of the magnetic moment associated with the electron's spin, and found the experimentally observed value, which was too large to be that of a spinning charged sphere governed by classical physics. He was able to solve for the spectral lines of the hydrogen atom, and to reproduce from physical first principles Sommerfeld's successful formula for the fine structure of the hydrogen spectrum.
Dirac's equations sometimes yielded a negative value for energy, for which he proposed a novel solution: he posited the existence of an antielectron and of a dynamical vacuum. This led to many-particle quantum field theory. In 1930, Dirac wrote the first modern textbook on quantum mechanics, one combining Heisenberg's matrix mechanics, Schrödinger's wave mechanics, and his own quantum transformation theory into a single presentation, while also encompassing theorizing based on relativity. The Principles of Quantum Mechanics was quickly recognized as a classic text, and remains valuable down to the present day.
Up to this point, all of quantum theory was mainly based on the atomic spectrum of the hydrogen atom. This is due to the fact that when the light emitted by a pure element is passed through a prism, the resulting pattern of spectral lines is unique to that element. Scientists could not study the electron and nucleus of the atom itself because they cannot be seen. Even today with the high-resolution Scanning Electron Microscope, the atom resolves to no more than a blurry fuzzball. However, the emission spectrum of an element reveal its electron orbitals and the energy differences between any two orbitals. The main empirical evidence for quantum theory thus far was the observed emission spectra of hydrogen and helium, and the mathematical formulas were made to fit these spectra. Hence quantum mechanics is sometimes referred to as a form of mathematical physics.
[edit] Quantum entanglement
Albert Einstein rejected Heisenberg's Uncertainty Principle insofar as it seemed to imply more than a necessary limitation on human ability to actually know what occurs in the quantum realm. In a letter to Max Born in 1926, Einstein famously declared that "God does not play dice with the universe."[34]
The bare surface level prescription for making predictions from quantum mechanics, based on Born's rule for computing probabilities, became known as the Copenhagen Interpretation of quantum mechanics. Bohr spent many years developing and refining this interpretation, keeping Einstein's objections in mind. After the 1930 Solvay conference, Einstein never again challenged the Copenhagen interpretation on mere technical grounds. But he did not cease his philosophical attack on that interpretation, on the grounds that quantum mechanics implied action at a distance, which is implausible in classical physics.
In trying to show that quantum theory was not a complete theory, Einstein started from that fact that the theory predicts that two or more particles that have interacted in the past can appear strongly correlated when their various properties are later measured. He sought to explain this seeming interaction in a classical way, through their common past, and preferably not by some "spooky action at a distance." The argument is worked out in a famous paper, Einstein, Podolsky, and Rosen (1935; abbreviated EPR), setting out what is now called the EPR paradox. Assuming what is now usually called local realism, EPR attempted to show from quantum theory that a particle has both position and momentum simultaneously, while according to the Copenhagen interpretation, only one of those two properties actually exists and only at the moment that it is being measured. EPR concluded that quantum theory is incomplete in that it refuses to consider physical properties which objectively exist in nature. (Einstein, Podolsky, & Rosen 1935 is currently Einstein's most cited publication in physics journals.)
The feature of quantum theory leading to these paradoxes is now called quantum entanglement. Quantum entanglement refers to situations where the properties of several discrete objects cannot be described simply by considering them separately, even after taking account of the history of how the objects have interacted in the past. A quantum interaction (such as the passage of a photon through a polarizer) can affect the entangled quantum entity in a similar manner, even though the two of them are widely separated.
Bohr's original response to Einstein was that entangled particles are part of an indivisible system. Einstein's challenge led to decades of research into quantum entanglement, which has confirmed Bohr's assertion that entangled particles must be viewed as one whole, and that difficulties arise only if one insists on the reality of outcomes of measurements not made. It appears that God does indeed throw dice, although rather peculiar ones. A real dice throw can be completely understood using the laws of classical mechanics, and the outcome is merely a function of the initial conditions. However the outcome of tossing quantum "dice" has no antecedent; it has as yet no discernible cause.
[edit] Interpretations
Quantum theory is without rival in its ability to explain known empirical phenomena, to predict new empirical findings, and to subsume other theories. The empirical predictions of quantum theory (especially quantum electrodynamics) have been confirmed to more significant digits than those of any other nontrivial scientific theory. Moreover, all modern fundamental physical theories, even special relativity, are quantum field theories. Nearly all of classical physics is now seen as a special case of quantum physics and/or relativity theory. Classical physics, however, also deals with gravity, a fundamental force of nature causing all masses to be attracted to each other, and there is as yet no quantum field treatment of general relativity, the accepted theory of gravitation. A single theory combining general relativity with relativistic quantum mechanics is the elusive Holy Grail of contemporary theoretical physics.
Notwithstanding its predictive success, some aspects of QM are counterintuitive. For example, QM attributes to microscopic objects a behavior very different from how we expect objects to behave, given our everyday macroscopic experience.
Interestingly, the correspondence principle and Ehrenfest's theorem predict that as a system becomes larger or more massive (so that action >> h ), classical dynamics tends to emerge (with some exceptions, such as superfluidity and superconductivity). Hence we can usually ignore QM when thinking about and manipulating everyday objects, and the classical description of such objects suffices. Even so, trying to make sense of QM has spawned a number of interpretations of quantum theory, ranging from the canonical Copenhagen Interpretation to the more radical hidden variables and many worlds interpretations.
[edit] See also
Persons important for discovering and elaborating quantum theory:
[edit] Further reading
The following titles, all by working physicists, attempt to communicate quantum theory to lay people, using a minimum of technical apparatus.
- Richard Feynman, 1985. QED: The Strange Theory of Light and Matter, Princeton University Press. ISBN 0-691-08388-6
- Ghirardi, GianCarlo, 2004. Sneaking a Look at God's Cards, Gerald Malsbary, trans. Princeton Univ. Press. The occasional passages using algebra, trigonometry, and bra-ket notation can be passed over on a first reading.
- Victor Stenger, 2000. Timeless Reality: Symmetry, Simplicity, and Multiple Universes. Buffalo NY: Prometheus Books. Includes much non-quantum physics and philosophy.
[edit] References
- Bernstein, Jeremy, 2005, "Max Born and the quantum theory," Am. J. Phys. 73(11).
- Beller, Mara, 2001. Quantum Dialogue: The Making of a Revolution. University of Chicago Press.
- Bohr, Niels (1958). Atomic Physics and Human Knowledge. John Wiley and Sons. OCLC 530611 ASIN B00005VGVF.
- Louis de Broglie, 1953. The Revolution in Physics. Noonday Press.
- Albert Einstein, 1934. Essays in Science. Philosophical Library.
- Herbert Feigl and May Brodbeck, 1953. Readings in the Philosophy of Science, Appleton-Century-Crofts.
- Fowler, Michael, 1999. The Bohr Atom. Lecture series, University of Virginia.
- Werner Heisenberg, 1958. Physics and Philosophy. Harper and Brothers.
- Lakshmibala, S., 2004, "Heisenberg, Matrix Mechanics and the Uncertainty Principle," Resonance, Journal of Science Education 9(8).
- Richard L. Liboff, 1992. Introductory Quantum Mechanics, 2nd ed.
- Lindsay, Robert Bruce and Henry Margenau, 1936. Foundations of Physics. Dover.
- McEvoy, J.P., and Zarate, Oscar. Introducing Quantum Theory, ISBN 1874166374
- Nave, Carl Rod, 2005. Hyperphysics-Quantum Physics, Department of Physics and Astronomy, Georgia State University, CD.
- Peat, F. David, 2002. From Certainty to Uncertainty: The Story of Science and Ideas in the Twenty-First Century. Joseph Henry Press.
- Hans Reichenbach, 1944. Philosophic Foundations of Quantum Mechanics. University of California Press.
- Paul Arthur Schilpp, 1949. Albert Einstein: Philosopher-Scientist. Tudor Publishing Company.
- Scientific American Reader, 1953.
- Sears, Francis Weston, 1949. Optics. Addison-Wesley.
- Shimony, A. (1983). "(title not given in citation)". Foundations of Quantum Mechanics in the Light of New Technology (S. Kamefuchi et al., eds.): p.225, Tokyo: Japan Physical Society.; cited in: Popescu, Sandu; Daniel Rohrlich. "Action and Passion at a Distance: An Essay in Honor of Professor Abner Shimony". arXiv.org. http://arxiv.org/abs/quant-ph/9605004. Retrieved on 2007-01-12.
- Takada, Kenjiro, Emeritus professor of Kyushu University, "Microscopic World-Introduction to Quantum Mechanics."
- "Uncertainty Prirnciple" Werner Heisenberg actual voice recording, http://www.thebigview.com/spacetime/index.html.
- Van Vleck, J. H.,1928, "The Correspondence Principle in the Statistical Interpretation of Quantum Mechanics," Proc. Nat. Acad. Sci. 14: 179.
- Veltman, M. J. G., 2003. Facts and Mysteries in Elementary Particle Physics. World Scientific Publishing Company.
- Wieman, Carl, and Perkins, Katherine, 2005, "Transforming Physics Education," Physics Today.
- Westmoreland, M. D., and Schumacher, B., 1998, "Quantum Entanglement and the Nonexistence of Superluminal Signals."
[edit] Notes
- ^ Huygens' principle is explained in Sears, Francis Weston, 1949. Optics. Addison-Wesley, pp. 5f.
- ^ Sears, Optics, p. 2f.
- ^ See Microwave Theory and Techniques, IEEE Transactions on 36(5): 830-58. ISSN:0018-9480.
- ^ Max Planck, 1901, "Über das Gesetz der Energieverteilung im Normalspectrum," Ann. Physik 4: 553. The events that led Planck to write it, and to deliver it as a speech in December 1900, are related by Werner Heisenberg in his Physics and Philosophy, pp. 30f. Heisenberg added that he believed that Planck was aware that his ideas would have far-reaching consequences.
- ^ Remarkably, in the same year (1905) Einstein published his landmark paper on special relativity. Thus Einstein helped pioneer quantum mechanics as well as discover special and general relativity. Einstein and Planck occasionally played tennis, although they spent much of their time discussing the emerging quantum mechanics! Richard Feynman noted Einstein's important contributions to QM as follows: "[The] phenomenon of 'stimulated emission' was discovered by Einstein when he launched the quantum theory proposing the photon model of light. Lasers work on the basis of this phenomenon." (Feynman, R., 1985. QED: The Strange Theory of Light and Matter. Princeton Univ. Press: 112.
- ^ Of Bohr's contributions to the quantum revolution, Einstein wrote: "[we] will have to connect one of the most important advances ever made in our knowledge of the nature of the atom with the name of Niels Bohr." He added, "The boldly selected hypothetical basis of his speculations soon became a mainstay for the physics of the atom... The theory of the Röntgen spectra of the visible spectra, and the periodic system of the elements are primarily based on the ideas of Bohr." (Einstein, A., Essays in Science, p. 46f.
- ^ Bohr noted De Broglie's contributions toward "a more comprehensive quantum theory" taking into account that "...the wave-corpuscle duality was not confined to the properties of radiation, but was equally unavoidable in accounting for the behaviour of material particles." (Bohr, N., Atomic Physics and Human Knowledge, p. 37 et passim.)
- ^ Born, M., Atomic Physics, especially p. 90, where he says of QM that it is "in the nature of the case indeterministic, and therefore the affair of statistics."
- ^ A two-page account of the highlights of Dirac's work, including his prediction of the positron, is in Gray, George W., 1953, "The Ultimate Particles" in The Scientific American Reader. Simon and Schuster: 100f.
- ^ Heisenberg is well known for his "indeterminacy principle" or uncertainty principle.
- ^ Reputed for discovering the Pauli Exclusion Principle, according to which it is impossible, in the words of Louis de Broglie, "for two electrons to have rigorously identical quantized states, i.e., defined by the same quantum numbers... Translated into wave mechanics, Pauli's principle is expressed as follows: 'for electrons, the only states realized in nature are the antisymmetric states.'" (De Broglie, L., The Revolution in Physics, p. 267.)
- ^ Schrödinger's cat was originally a fictional character in an example Schrodinger thought up to criticise an apparent difficulty in Heisenberg's exposition of his uncertainty principle. The story has been taken somewhat out of context and the cat has assumed a minor literary life of its own. Schrödinger's purely technical contributions to QM and to making its mathematics easier to handle are, of course, much more important. Here is a translation of his 1935 essay introducing the proverbial cat. Schrödinger describes a situation in which a cat will live or die depending on whether a quantum mechanically probabilistic radioactive emission event occurs within the hour that the cat is confined to a box. To Heisenberg's interpretation of QM he objected: "If one has left this entire system to itself for an hour, one would say that the cat still lives if meanwhile no atom has decayed. The psi-function of the entire system would express this by having in it the living and dead cat (pardon the expression) mixed or smeared out in equal parts."
- ^ Lindsay and Margenau, Foundations of Physics, p. 388}}
- ^ Sears, Mechanics, Wave Motion, and Heat, p. 537.
- ^ A. Einstein, Ann. d. Phys., 17, 132, (1905).
- ^ Dicke and Wittke, Introduction to Quantum Mechanics, p. 12
- ^ A very clear explanation of interference in thin films may be found in Sears, op. cit., p. 203ff.
- ^ J. P. McEvoy and Oscar Zarate, Introducing Quantum Theory, pp. 114, 118.
- ^ A. P. French and Edwin F. Taylor, An Introduction to Quantum Physics,, p. 18.
- ^ Robert H. Dicke and James P. Wittke, 1960. Introduction to Quantum Mechanics. Addison-Wesley: 9f.
- ^ For the length of time involved, see George Gamow's One, Two, Three...Infinity, p. 140.
- ^ Dicke and Wittke, "Introduction to Quantum Mechanics, p. 10f.
- ^ A. P. French and Edwin F. Taylor, An Introduction to Quantum Physics,, p. 23.
- ^ See Linus Pauling, The Nature of the Chemical Bond,
- ^ Werner Heisenberg, "Quantum-Theoretical Re-interpretations of Kinematic and Mechanical Relations," in B. L. van der Waerden, ed., Sources of Quantum Mechanics. Dover Publications: p. 266.
- ^ Aitchison, I.J.R., "Understanding Heisenberg's 'magical' paper of July 1925: a new look at the calculational details," explicates these calculations in detail.
- ^ Lakshmibala, S., 2004, "Heisenberg, Matrix Mechanics, and the Uncertainty Principle," Resonance 9(8): 48f.
- ^ Z. Phys. 33: 879-93. It has been translated and reprinted in B.L. van der Waerden, 1968. Sources of Quantum Mechanics. Dover Publications.
- ^ Aitchison, I.J.R., "Understanding Heisenberg's 'magical' paper of July 1925: a new look at the calculational details," p. 5
- ^ W. Moore, Schrödinger: Life and Thought, Cambridge University Press (1989), p. 222.
- ^ Quantum Mechanics 1925-1927: Sound Bites
- ^ Another way to say this is that the square of the amplitude (the wave intensity) gives the probability of finding a photon at the corresponding point along that wavefront. See Dicke and Wittke, Introduction to Quantum Mechanics, p. 22.
- ^ a b Linus Pauling, The Nature of the Chemical Bond, p. 47
- ^ Letter from A. Einstein to M. Born dated December 12, 1926, as reproduced in Born, M., 1969. Physics in my generation. Springer-Verlag: p. 113. Because Einstein has been often misquoted, we give the two original German sentences: "Die Theorie liefert viel, aber dem Geheimnis des Alten bringt sie uns kaum näher. Jedenfalls bin ich überzeugt, daß der nicht würfelt." Born's translation: "The theory yields much, but it hardly brings us nearer to the secret of the Old One. In any case, I am convinced that he does not throw dice."
[edit] External links
- Takada, Kenjiro, Emeritus professor at Kyushu University, "Microscopic World -- Introduction to Quantum Mechanics."
- Westmoreland, M. D., and Schumacher, B., 1998, "Quantum Entanglement and the Nonexistence of Superluminal Signals."
- Quantum Theory.
- Quantum Mechanics.
- Planck's original paper on Planck's constant.
- Everything you wanted to know about the quantum world. From the New Scientist.
- Quantum Articles.
- This Quantum World.
- The Quantum Exchange (tutorials and open source learning software).
- Theoretical Physics wiki
- "Uncertainty Principle," a recording of Werner Heisenberg's voice.












