Bohm interpretation

From Wikipedia, the free encyclopedia

The Bohm or Bohmian interpretation of quantum mechanics, which Bohm called the causal, or later, the ontological interpretation, is an interpretation postulated by David Bohm in 1952 as an alternative to the standard Copenhagen interpretation. The Bohm interpretation grew out of the search for an alternative model based on the assumption of hidden variables. Its basic formalism corresponds in the main to Louis de Broglie's pilot-wave theory of 1927. Consequently it is sometimes called the de Broglie-Bohm theory. The interpretation was developed further during the sixties and seventies under the heading of the causal interpretation in order to distinguish it from the purely probabilistic approach of the standard interpretation. Bohm later extended the approach to include both a deterministic and a stochastic version. The fullest presentation is given in Bohm and Hiley The Undivided Universe, presented under the heading of an ontological interpretation to emphasize its concern with “beables” rather than with “observables,” and in contradistinction to the predominantly epistemological approach of the standard model. In its final form, building on the insights of Bell and others, the ontological interpretation is causal but non-local, and non-relativistic, while capable of being extended beyond the domain of the current quantum theory in a number of ways.

In the Bohm interpretation, every particle has a definite position and momentum at all times, but we do not usually know what they are, though we do have limited information about them. The particles are guided by the wave function, which follows the Schrödinger equation.

The Bohm interpretation is an example of a hidden variables theory. Bohm originally hoped that hidden variables could provide a local, causal, objective description that would resolve or eliminate many of the paradoxes of quantum mechanics, such as Schrödinger's cat, the measurement problem and the collapse of the wavefunction. However, Bell's theorem complicates this hope, as it demonstrates that there is no locally causal hidden variable theory that is compatible with quantum mechanics. The Bohmian interpretation is causal but not local.

The Bohm interpretation is non-relativistic.

The Bohm interpretation is an interpretation of quantum mechanics. In other words, it has not been disproven, but there are other schemes (such as the Copenhagen interpretation) that give the same theoretical predictions, so are equally confirmed by the experimental results.

[edit] The theory

[edit] Principles

The Bohm interpretation is based on these principles:

Every particle travels in a definite path

Each particle is viewed as having a definite position and velocity at all times.

We do not know what that path is

Measurements allow us to successively retroactively refine the bounds on the particle path at some time, but there always remains some classical uncertainty in the position and momentum (as there always is in any classical theory), which increases with time.

Every particle is accompanied by a field, which guides the motion of the particle

De Broglie called this the pilot wave; Bohm called it the ψ-field. This field has a piloting influence on the motion of the particles. The quantum potential is derived from the ψ-field.

This field satisfies the Schrödinger equation

Mathematically, the field corresponds to the wavefunction of conventional quantum mechanics, and evolves according to the Schrödinger equation. The positions of the particles do not affect the wave function.

The particle's momentum p is $\nabla S(x,t)$

The particles momentum can be calculated from the value of the wavefunction at the position of the particle. See the section on One-particle formalism for a discussion of the mathematics.

The particles form a statistical ensemble, with probability density $\rho(\mathbf{x},t) = |\psi(\mathbf{x},t)|^2$

Although we don't know the position of any individual particle before we measure them, we find after the measurement that the statistics conform to the probability density function that is based on the wavefunction in the usual way.

In its basic form, the Bohm interpretation is non-relativistic; it does not attempt to deal with high speeds or significant gravity. There are extensions that address relativistic issues.

The Bohm interpretation is an interpretation of quantum mechanics; it was originally developed as an objective and deterministic alternative to the Copenhagen interpretation. It says that the state of the universe evolves smoothly through time, with no collapsing of wavefunctions.

The Bohm interpretation is a hidden variables theory. In other words, there is a precisely defined history of the universe; however, some of the variables that define the history are not (and cannot be) known to the observer. For that reason, there is uncertainty in what we know about the universe.

[edit] Name and evolution

The Bohm Interpretation is not a single closed theory, but is open-ended and has evolved through stages. Occasionally, to understand a paper, it is necessary to identify the stage that it refers to.

In this section, each stage is given a name and a main reference. To get an understanding of which stage a paper refers to, the name is a quick (but unreliable) clue; comparing the references gives a better understanding.

Pilot-wave theory

This was the theory which de Broglie presented at the 1927 Solvay Conference^[1].

This stage applies to many spin-less particles, and is deterministic, but lacks an adequate theory of measurement.

De Broglie-Bohm Theory or Bohmian Mechanics

This was described by Bohm's original papers 'A Suggested Interpretation of the Quantum Theory in Terms of "Hidden Variables" I and II' [Bohm 1952]. It extended the original Pilot Wave Theory to incorporate a consistent theory of measurement, and to address a criticism of Pauli that de Broglie did not properly respond to^{[clarification needed]}; it is taken to be deterministic (though Bohm hinted in the original papers that there should be disturbances to this, in the way Brownian motion disturbs Newtonian mechanics). This stage is known as the de Broglie-Bohm Theory in Bell's work [Bell 1987] and is the basis for 'The Quantum Theory of Motion' [Holland 1993]. It is also referred to in some papers as Bohmian Mechanics.

This stage applies to multiple particles, and is considered by most authors to be deterministic.

Causal Interpretation and Ontological Interpretation

Bohm developed his original ideas, calling them the Causal Interpretation. Later he felt that causal sounded too much like deterministic and preferred to call his theory the Ontological Interpretation. The main reference is 'The Undivided Universe' [Bohm, Hiley 1993].

These stages cover work by Bohm and in collaboration with Vigier and Hiley. Bohm is clear that this theory is non-deterministic (the work with Hiley includes a stochastic theory).

As an example, take the paper "A first experimental test of de Broglie-Bohm theory against standard quantum mechanics" ^[2] This refers to "On the Incompatibility of Standard Quantum Mechanics and the de Broglie-Bohm Theory"[2]. These papers base their argument on determinism, and refer to [Holland 1993] as the reference. One can see that they refer to the deterministic de Broglie-Bohm theory but not to Bohm's Causal interpretation.

[edit] Results

The Bohm interpretation demonstrates that some features of the Copenhagen interpretation are not essential to Quantum mechanics, but are a feature of the interpretation. Some specific features are:

wave collapse
entanglement
the non-existence of particles while not being observed

Examination of the Bohm interpretation has shown that nonlocality is a general feature of Quantum mechanics interpretations, including the Copenhagen interpretation where it was not originally obvious.

[edit] Reformulating the Schrödinger equation

Some of Bohm's insights are based on a reformulation of the Schrödinger equation; instead of using the wavefunction $\psi(\mathbf{x},t)$ , he solves it for the magnitude $R(\mathbf{x},t)$ and complex phase $S(\mathbf{x},t)$ of the wavefunction. This section presents the mathematics; the next section presents the insights.

The Schrödinger equation for one particle of mass m is

$i \hbar \frac{\partial \psi(\mathbf{x},t)}{\partial t} = \frac{-\hbar^2}{2 m} \nabla^2 \psi(\mathbf{x},t)+ V(\mathbf{x}) \psi(\mathbf{x},t)$ ,

where the wavefunction $\psi(\mathbf{x},t)$ is a complex function of the spatial coordinate $\mathbf{x}$ and time t.

The probability density 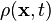$\rho (\mathbf{x},t)$ is a real function defined as the magnitude of the wave function:

$\rho(\mathbf{x},t) = |\psi(\mathbf{x},t)|^2$ .

We can express the wavefunction $\psi(\mathbf{x}, t)$ in polar coordinates; Without loss of generality, we can define real functions $R(\mathbf{x},t)$ and $S(\mathbf{x},t)$ such that:

$\psi(\mathbf{x},t) = R(\mathbf{x},t)e^{i S(\mathbf{x},t) / \hbar}$ .

The Schrödinger equation can then be split into two coupled equations by expressing it in terms of R and S:

$\frac{\partial R(\mathbf{x},t)}{\partial t} = \frac{-1}{2m}[R(\mathbf{x},t)\nabla ^2S(\mathbf{x},t)+2\nabla R(\mathbf{x},t)\cdot \nabla S(\mathbf{x},t)]$

$\frac{\partial S(\mathbf{x},t)}{\partial t} = -\left[ V + \frac{1}{2m}(\nabla S(\mathbf{x},t))^2 -\frac{\hbar ^2}{2m} \frac{\nabla ^2R(\mathbf{x},t)}{R(\mathbf{x},t)} \right]$

The magnitude $R(\mathbf{x},t)$ is $|\psi (\mathbf{x},t)|$ , so that $R^2(\mathbf{x},t)$ corresponds to the probability density $\rho (\mathbf{x},t) = |\psi (\mathbf{x},t)|^2$ .

$S(\mathbf{x},t)$ is the complex phase chosen to have the units and typical variable name of an action. Thus

$\psi(\mathbf{x},t) = \sqrt{\rho(\mathbf{x},t)} e^{i S(\mathbf{x},t) / \hbar}$ .

Therefore we can substitute 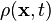$\rho(\mathbf{x},t)$ for $R^2(\mathbf{x},t)$ and get:

$-\frac{\partial \rho(\mathbf{x},t)}{\partial t} = \nabla \cdot \left(\rho (\mathbf{x},t)\frac{\nabla S(\mathbf{x},t)}{m}\right) \qquad (1)$

$-\frac{\partial S(\mathbf{x},t)}{\partial t} = V(\mathbf{x}) + Q(\mathbf{x},t) + \frac{1}{2m}(\nabla S(\mathbf{x},t))^2 \qquad (2)$

where

$Q(\mathbf{x},t) = -\frac{\hbar^2}{2 m} \frac{\nabla^2 R(\mathbf{x},t)}{R(\mathbf{x},t)} = -\frac{\hbar^2}{2 m} \frac{\nabla^2 \sqrt{\rho(\mathbf{x},t)}}{ \sqrt{\rho(\mathbf{x},t)}} = -\frac{\hbar^2}{2 m} \left(\frac{\nabla^2 \rho(\mathbf{x},t)}{2 \rho(\mathbf{x},t)} -\left(\frac{\nabla \rho(\mathbf{x},t)}{2 \rho(\mathbf{x},t)} \right)^2 \right)$

Bohm called the function $Q(\mathbf{x},t)$ the quantum potential.

We can use the same argument on the many-particle Schrödinger equation:

$i \hbar \frac{\partial \psi(\mathbf{x_1,x_2,...},t)}{\partial t} = \sum_i \frac{-\hbar^2}{2 m_i} \nabla_i^2 \psi(\mathbf{x_1, x_2,..},t) + V(\mathbf{x_1, x_2,..})\psi(\mathbf{x_1, x_2,...},t)$ ,

where the i-th particle has mass 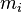$m_i\,$ and position coordinate $\mathbf{x_i}$ at time t. The wavefunction $\psi(\mathbf{x_1, x_2,...},t)$ is a complex function of all the $\mathbf{x_i}$ and time t. $\nabla_i$ is the grad operator with respect to $\mathbf{x_i}$ , i.e. of the i-th particle's position coordinate. As before the probability density $\rho(\mathbf{x_1, x_2,...},t)$ is a real function defined by

$\rho(\mathbf{x_1, x_2,..},t) = |\psi(\mathbf{x_1, x_2,..},t)|^2$ .

As before, we can define a real function $S(\mathbf{x_1, x_2,...},t)$ to be the complex phase,so that we can define a similar relationship to the 1-particle example:

$\psi(\mathbf{x_1, x_2,..},t) = \sqrt{\rho(\mathbf{x_1, x_2,..},t)} e^{i S(\mathbf{x_1, x_2,..},t) / \hbar}$ .

We can use the same argument to express the Schrödinger equation in terms of $\rho(\mathbf{x_1, x_2,..},t)$ and $S(\mathbf{x_1, x_2,..},t)$ :

$-\frac{\partial \rho(\mathbf{x_1, x_2,..},t)}{\partial t} = \sum_i \nabla_i \cdot (\rho(\mathbf{x_1, x_2,..},t) \frac{\nabla_i S(\mathbf{x_1, x_2,..},t)}{m_i}) \qquad (3)$

$-\frac{\partial S(\mathbf{x_1, x_2,..},t)}{\partial t} = V(\mathbf{x_1, x_2,..}) + Q(\mathbf{x_1, x_2,..},t) + \sum_i \frac{1}{2m_i}(\nabla_i S(\mathbf{x_1, x_2,..},t))^2 \qquad (4)$

where

$Q(\mathbf{x_1, x_2,..},t) = -\sum_i \frac{\hbar^2}{2 m_i} \frac{\nabla_i^2 R(\mathbf{x_1, x_2,..},t)}{R(\mathbf{x_1, x_2,..},t)} = -\sum_i\frac{\hbar^2}{2 m_i} \left(\frac{\nabla_i^2 \rho(\mathbf{x_1, x_2,..},t)}{2 \rho(\mathbf{x_1, x_2,..},t)} -\left(\frac{\nabla_i \rho(\mathbf{x_1, x_2,..},t)}{2 \rho(\mathbf{x_1, x_2,..},t)} \right)^2 \right)$ .

[edit] One-particle formalism

In his 1952 paper, Bohm starts from the reformulated Schrödinger equation. He points out that in equation (2):

$-\frac{\partial S(\mathbf{x},t)}{\partial t} = V(\mathbf{x}) + Q(\mathbf{x},t) + \frac{1}{2m}(\nabla S(\mathbf{x},t))^2$ ,

if one represents the world of classical physics by setting $\hbar$ to zero (which results in Q becoming zero) then $S(\mathbf{x},t)$ is the solution to the Hamilton-Jacobi equation. He quotes a theorem that says that if an ensemble of particles (which follow the equations of motion) have trajectories that are normal to a surface of constant S, then they are normal to all surfaces of constant S, and that $\nabla S(\mathbf{x},t)/m$ is the velocity of any particle passing point $\mathbf{x}$ at time t.

Therefore, we can express equation (1) as:

$-\frac{\partial \rho(\mathbf{x},t)}{\partial t} = \nabla \cdot \left(\rho (\mathbf{x},t)\mathbf{v}\right)$

This equation shows that it is consistent to express 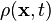$\rho (\mathbf{x},t)$ as the probability density because $\rho (\mathbf{x},t)\mathbf{v}$ is then the mean current of particles, and the equation expresses the conservation of probability.

Of course, $\hbar$ is non-zero. Bohm suggests that we still treat the particle velocity as $\nabla S(\mathbf{x},t)/m$ . The movement of a particle is described by equation (2):

$-\frac{\partial S(\mathbf{x},t)}{\partial t} = V(\mathbf{x}) + Q(\mathbf{x},t) + \frac{1}{2m}(\nabla S(\mathbf{x},t))^2$

where

$Q(\mathbf{x},t) = -\frac{\hbar^2}{2 m} \left(\frac{\nabla^2 \rho(\mathbf{x},t)}{2 \rho(\mathbf{x},t)} -\left(\frac{\nabla \rho(\mathbf{x},t)}{2 \rho(\mathbf{x},t)} \right)^2 \right)$

V is the classical potential, which influences the particle's movement in the ways described by the classical laws of motion.

Q also has the form of a potential; it is known as the quantum potential. It influences particles in ways that are specific to quantum theory. Thus the particle is moving under the influence of a quantum potential Q as well as the classical potential V.

The quantum potential does not vary with the strength of the ψ-field; this is evident because ρ and its derivatives appear both in the numerator and the denominator of the quantum potential. Thus the quantum potential guides the particles, even when the ψ-field is weak.

[edit] Many-particle formalism

The momentum of Bohm's i-th particle's "hidden variable" is defined by

$\mathbf{p_i} = {m_i} \mathbf{v_i} = \nabla_i S \qquad (3)$

and the particles' total energy as $E = - \partial S / \partial t$ ; equation (1) is the continuity equation for probability with

$\mathbf{j_i} = \rho \mathbf{v_i} = \rho \frac{\mathbf{p_i}}{m_i} = \rho \frac{\nabla_i S}{m_i}$ ,

and equation (4) is a statement that total energy is the sum of the potential energy, quantum potential and the kinetic energies.

[edit] Comparison with experimental data

An important prediction of the Bohm theory, made in Bohm's original 1952 paper, is that the electron in the ground state of a hydrogen atom is in rest (cf. equation (3) above - s states have spherical symmetry and thus have constant phase), as the quantum force introduced by Bohm balances the classical electromagnetic potential.

Any measurement of the momentum of a ground state electron will give a non-zero result as predicted by quantum mechanics, but Bohm's theory argues that the act of measuring the momentum disturbs the electron at rest, resulting in a non-zero expection value. ^[3]

Experimental observation of the decay rates of muons bound in exotic atoms has shown, however, that ground state electrons are in fact in motion. Because of their mass, muons captured in higher states rapidly cascade to the ground state, and about 99 percent of the bound muons decay from the 1S state. If the atomic number of the hydrogen-like atom is high enough, the muon motion will be relativistic, and subject to time dilation. The data show that for moderate Z atoms, the observed lengthening of the muon decay time can be attributed to the relativistic time dilation.^[4]

Since the motion of the muon has been demonstrated without any disturbance of the atom, it cannot be explained by disturbances related to the measurement process as with conventional measurements of the momentum of ground state electrons. An important conceptual prediction of the Bohm model, namely that ground state electrons are at rest, would seem to be contradicted by experimental evidence. But Bohm's prediction of the lack of motion of the lepton in the s state is only a non-relativistic prediction, since the Schrödinger equation is non-relativistic. Therefore the muon result, which relies on time dilation, is not at variance with the accepted theoretical demonstration that Bohm's theory reproduces all the non-relativistic results of the other conventional quantum interpretations.

Further, it should be noted that since relativistic quantum theories (such as quantum field theory) can always be expressed in terms of a local Lagrangian density, it follows that probability mass in such theories always flows locally through configuration space, and therefore that a classical configuration of the system's (field) variables can still be made to evolve locally in a way that simply tracks the flow of the conserved probability current in configuration space. Therefore, Bohm's interpretation can be extended to a relativistic version that works in such a way that it exactly duplicates the predictions of standard quantum field theory, so, in fact, there can be no experimental contradiction of Bohm's approach (if suitably generalized in this way) that does not also contradict the standard model.^{[citation needed]}

[edit] Understanding quantum mechanics

[edit] Indeterminism in the Bohm Interpretation.

A major difference between Bohm’s interpretation and the usual one is the approach to the indeterminism. In the usual interpretation, the Schrödinger equation is treated not as an actual field, but as a probability density matrix (Born) which yields the resultant statistical data. According to the founding fathers, there is no way to produce results which would be more exact by referring to any “hidden variables” beyond the theory itself. Thus, in the usual interpretation, there is what Bohm calls an “irreducible lawlessness” which goes beyond our lack of knowledge or coarse graining.

For Bohm, on the other hand, the indeterminism implied by the theory is only at the level of the macroscopic experimental apparatus (e.g. the observables), and is not part of the nature of reality. Bohm expected that the underlying motions of a sub-quantum domain could be more precisely defined than allowed by the standard model, so that the degrees of freedom implied are considerably reduced.

[edit] Heisenberg's uncertainty principle

The Heisenberg uncertainty principle states that when two complementary measurements are made, there is a limit to the product of their accuracy. As an example, if one measures the position with an accuracy of $Δ x$ , and the momentum with an accuracy of $Δ p$ , then $\Delta x\Delta p\gtrsim h.$ If we make further measurements in order to get more information, we disturb the system and change the trajectory into a new one depending on the measurement set up; therefore, the measurement results are still subject to Heisenberg's uncertainty relation.

In Bohm's interpretation, there is no uncertainty in position and momentum of a particle; therefore a well defined trajectory is possible, but we have limited knowledge of what this trajectory is (and thus of the position and momentum). It is our knowledge of the particle's trajectory that is subject to the uncertainty relation. What we know about the particle is described by the same wave function that other interpretations use, so the uncertainty relation can be derived in the same way as for other interpretations of quantum mechanics.

To put the statement differently, the particles' positions are only known statistically. As in classical mechanics, successive observations of the particles' positions refine the initial conditions. Thus, with succeeding observations, the initial conditions become more and more restricted. This formalism is consistent with normal use of the Schrödinger equation. It is the underlying chaotic behaviour of the hidden variables that allows the defined positions of the Bohm theory to generate the apparent indeterminacy associated with each measurement, and hence recover the Heisenberg uncertainty principle.

For the derivation of the uncertainty relation, see Heisenberg uncertainty principle, noting that it describes it from the viewpoint of the Copenhagen interpretation.

[edit] Two-slit experiment

The double-slit experiment is an illustration of wave-particle duality. In it, a beam of particles (such as photons) travels through a barrier with two slits removed. If one puts a detector screen on the other side, the pattern of detected particles shows interference fringes characteristic of waves; however, the detector screen responds to particles. The photons must exhibit behaviour of both waves and particles.

The Copenhagen interpretation requires that the photons are not localised in space until they are detected, and travel through both slits.

In the Bohm interpretation, the guiding wave travels through both slits and sets up a ψ-field; the initial positions of the photons are not known to the observer, but they travel under the guidance of the ψ-field. Each photon that is detected has travelled through one of the slits; together, their statistics show the probability distribution of the ψ-field, which gives interference fringes that are characteristic of waves. The photons are localized particles at all times, and hence are able to activate the detector screen.

[edit] Measuring spin and polarization

It is not possible to measure the spin or polarization of a particle directly; instead, the component in one direction is measured; the outcome from a single particle may be 1, meaning that the particle is aligned with the measuring apparatus, or -1, meaning that it is aligned the opposite way. For an ensemble of particles, if we expect the particles to be aligned, the results are all 1. If we expect them to be aligned oppositely, the results are all -1. For other alignments, we expect some results to be 1 and some to be -1 with a probability that depends on the expected alignment. For a full explanation of this, see the Stern-Gerlach Experiment.

In the Bohm interpretation, we calculate the wave function that corresponds to the setup of the apparatus (including the orientation of the detectors); the particles are guided by this function, so we can calculate the probabilities that the actual particles will reach each part of the detector apparatus.

[edit] The Einstein-Podolsky-Rosen paradox

In the Einstein-Podolsky-Rosen paradox^[5], the authors point out that it is possible to create pairs of particles with quantum states that are mirror-images of each other; these particles are now described as entangled. They describe a thought-experiment showing that either quantum mechanics is an incomplete theory or that it has nonlocality.

John Bell then described Bell's theorem (see p.14 in^[6]), in which he shows that all hidden-variable theories (including the Bohm interpretation) have nonlocality. Bell went further, showing that quantum mechanics itself is nonlocal and that this cannot be avoided by appealing to any alternative interpretation (p. 196 in^[7]): "It is known that with Bohm's example of EPR correlations, involving particles with spin, there is an irreducible nonlocality."

Alain Aspect took this further by creating Bell test experiments, which realize the thought experiments on which Bell's theorem is based. He was able to show experimentally that Bell's results hold.

In the Bell test experiment, entangled pairs of particles are created; the particles are separated, travelling to remote measuring apparatus. The orientation of the measuring apparatus can be changed while the particles are in flight, demonstrating the non-locality of the effect. The apparatus makes a statistical detection of the orientation of the particles (see Measuring spin and polarization for a description of this). Bell's Theorem shows that the results at each detector depend on the orientation of both detectors.

The Bohm interpretation describes this experiment as follows: to understand the evolution of these particles, we need to set up a wave equation for both particles; the orientation of the apparatus affects the wave function. The particles in the experiment follow the guidance of the wave function; we don't know the initial conditions of these particles, but we can predict the statistical outcome of the experiment from the wave function. It is the wave function that carries the faster-than-light effect of changing the orientation of the apparatus.

[edit] History

Bohm became dissatisfied with the conventional interpretation of quantum mechanics, pointing out that, although it requires one to give up "the possibility of even conceiving what might determine the behaviour of an individual system at a quantum level", it doesn't prove that this requirement is necessary.

To highlight this, Bohm published 'A Suggested Interpretation of Quantum Mechanics in Terms of "Hidden" Variables' [Bohm 1952]. In this paper, Bohm presented his alternative.

While preparing this paper, Bohm became aware of de Broglie's Pilot wave theory. This was a hidden-variable theory which de Broglie presented at the 1927 Solvay Conference^[8]. At the conference, Wolfgang Pauli pointed out that it did not deal properly with the case of inelastic scattering. De Broglie was persuaded by this argument, and abandoned this theory. Later, in 1932, John von Neumann published a paper^[9], claiming to prove that all hidden-variable theories are impossible. This clearly applied to both de Broglie's and Bohm's theories.

Bohm's paper was largely ignored by other physicists; surprisingly, it was not supported by Albert Einstein (who was also dissatisfied with the prevailing orthodoxy and had discussed Bohm's ideas with him before publication). So Bohm lost interest in it.

The cause was taken up by John Bell. In "Speakable and Unspeakable in Quantum Mechanics" [Bell 1987], several of the papers refer to hidden variables theories (which include Bohm's). Bell showed that Pauli's and von Neumann's objections amounted to showing that hidden variables theories are nonlocal, and that nonlocality is a feature of all quantum mechanical systems.

The Bohm interpretation is now considered by some to be a valid challenge to the prevailing orthodoxy of the Copenhagen Interpretation, but it remains controversial.

[edit] Extensions

[edit] Exploiting Nonlocality

Antony Valentini of the Perimeter Institute has extended the Bohm Interpretation to include signal nonlocality that would allow entanglement to be used as a stand-alone communication channel without a secondary classical "key" signal to "unlock" the message encoded in the entanglement. This violates orthodox quantum theory but it has the virtue that it makes the parallel universes of the chaotic inflation theory observable in principle.

Valentini cites earlier work that shows that orthodox quantum theory corresponds to "sub-quantal thermal equilibrium" for the hidden variables.

The larger theory is a non-equilibrium theory: in Bohm's ontology, the hidden variable "particles" and "field configurations" receive their marching orders from the ψ-wave, but do not directly react back on it (see Ch 14 of the "Undivided Universe"); in Valentini's theory, this direct feedback of particle on its guiding pilot wave introduces an instability, a feedback loop that pushes the hidden variables out of thermal equilibrium "sub-quantal heat death" (Valentini). The resulting theory becomes nonlinear and non-unitary. The Born probability interpretation can no longer be sustained in this emergence of new order in complex systems that P.W. Anderson has called "More is different."

[edit] Isomorphism to the many worlds interpretation

Explicitly non-local. Bohm accepts that all the branches of the universal wavefunction exist. Like Everett, Bohm held that the wavefunction is real complex-valued field which never collapses. In addition Bohm postulated that there were particles that move under the influence of a non-local "quantum- potential" derived from the wavefunction (in addition to the classical potentials which are already incorporated into the structure of the wavefunction). The action of the quantum- potential is such that the particles are affected by only one of the branches of the wavefunction. (Bohm derives what is essentially a decoherence argument to show this, see section 7,#I [B]).

The implicit, unstated assumption made by Bohm is that only the single branch of wavefunction associated with particles can contain self-aware observers, whereas Everett makes no such assumption. Most of Bohm's adherents do not seem to understand (or even be aware of) Everett's criticism, section VI [1]^[10], that the hidden- variable particles are not observable since the wavefunction alone is sufficient to account for all observations and hence a model of reality. The hidden variable particles can be discarded, along with the guiding quantum-potential, yielding a theory isomorphic to many-worlds, without affecting any experimental results.

—^[11]

[edit] Quantum trajectory method

Work by Robert Wyatt in the early 2000s attempted to use the Bohm "particles" as an adaptive mesh that follows the actual trajectory of a quantum state in time and space. In the "quantum trajectory" method, one samples the quantum wave function with a mesh of quadrature points. One then evolves the quadrature points in time according to the Bohm equations of motion. At each time-step, one then re-synthesizes the wave function from the points, recomputes the quantum forces, and continues the calculation. (Quick-time movies of this for H+H₂ reactive scattering can be found on the Wyatt group web-site at UT Austin.) This approach has been adapted, extended, and used by a number of researchers in the Chemical Physics community as a way to compute semi-classical and quasi-classical molecular dynamics. A recent issue of the Journal of Physical Chemistry A was dedicated to Prof. Wyatt and his work on "Computational Bohmian Dynamics".

Eric Bittner's group at the University of Houston has advanced a statistical variant of this approach that uses Bayesian sampling technique to sample the quantum density and compute the quantum potential on a structureless mesh of points. This technique was recently used to estimate quantum effects in the heat-capacity of small clusters Ne_n for n~100.

There remain difficulties using the Bohmian approach, mostly associated with the formation of singularities in the quantum potential due to nodes in the quantum wave function. In general, nodes forming due to interference effects lead to the case where $\frac{1}{R}\nabla^2R\rightarrow\infty.$ This results in an infinite force on the sample particles forcing them to move away from the node and often crossing the path of other sample points (which violates single-valuedness). Various schemes have been developed to overcome this; however, no general solution has yet emerged.

There has also been recent work in developing Complex Bohmian trajectories which satisfy isochronal relations.

[edit] Quantum chaos

There are developments in quantum chaos. In this theory, there exist quantum wave functions that are fractal and thus differentiable nowhere. While such wave functions can be solutions of the Schrödinger equation, taken in its entirety, they would not be solutions of Bohm's coupled equations for the polar decomposition of ψ into ρ and S, (see Reformulating the Schrödinger equation). The breakdown occurs when expressions involving ρ or S become infinite (due to the non-differentiability), even though the average energy of the system stays finite, and the time-evolution operator stays unitary. As of 2005^[update], it does not appear that experimental tests of this nature have been performed.

[edit] Other extensions

The theory can also easily be extended to include spin, as well as the relativistic Dirac theory. In the latter case, such relativistic extensions inherently involve an experimentally unobservable preferred frame, and this is considered by many to be in tension with the "spirit" of special relativity.

As probability density and probability current can be defined for any set of commuting operators, the Bohmian formalism is not limited to the position operator.^[12]

[edit] Frequently asked questions

Q: Why should we consider this to be a separate theory when it looks contrived, and gives the same measurable predictions as conventional quantum mechanics?

A: Bohm's original aim was to demonstrate that hidden-variables theories are possible, and his hope was that this demonstration could lead to new insights, measurable predictions and experiments.^[13] So far, the Bohm interpretation has had these results:

it has shown that a hidden-variable theory is possible; this has highlighted that some parts of other interpretations (such as wave collapse) are part of the interpretation and not an inevitable part of physics.
it has highlighted the issue of nonlocality: it inspired John Stewart Bell to prove his now-famous theorem,^[14] which in turn led to the Bell test experiments. These showed that all theories of quantum mechanics must address nonlocality.

Q: While orthodox quantum mechanics admits many observables on the Hilbert space that are treated almost equivalently (much like the bases composed of their eigenvectors), Bohm's interpretation requires one to pick a set of "privileged" observables that are treated classically — namely the position. How can this be justified, when there is no experimental reason to think that some observables are fundamentally different from others?

A: Positions may be considered as a natural choice for the selection because positions are most directly measurable. For example, one does not actually measure the "spin" of a particle in the Stern–Gerlach experiment, but instead measures the position of the light flashes on a detector. Often the observed quantities are positions, e.g. of a measuring needle or of the particles making up a computer display. And so there is justification for making position privileged.

Q: The Bohmian models are nonlocal; how can this be reconciled with the principles of special relativity? they make it highly nontrivial to reconcile the Bohmian models with up-to-date models of particle physics, such as quantum field theory or string theory, and with some very accurate experimental tests of special relativity, without some additional explanation. On the other hand, other interpretations of quantum mechanics, such as consistent histories or the many-worlds interpretation, allow us to explain the experimental tests of quantum entanglement without any nonlocality whatsoever.

A: It is questionable whether other interpretations of quantum theory are local or are simply less explicit about nonlocality. See for example the EPR type of nonlocality. And recent tests of Bell's Theorem add weight to the belief that all quantum theories must abandon either the principle of locality or counterfactual definiteness (the ability to speak meaningfully about the definiteness of the results of measurements, even if they were not performed).

Finding a Lorentz-invariant expression of the Bohm interpretation (or any nonlocal hidden-variable theory) has proved difficult, and it remains an open question for physicists today whether such a theory is possible and how it would be achieved.

There has been work in this area. See Bohm and Hiley: The Undivided Universe, and [3], [4], and references therein.

Q: The wavefunction must "disappear" or "collapse" after a measurement, and this process seems highly unnatural in the Bohmian models. The Bohmian interpretation also seems incompatible with modern insights about decoherence that allow one to calculate the "boundary" between the "quantum microworld" and the "classical macroworld"; according to decoherence, the observables that exhibit classical behavior are determined dynamically, not by an assumption.

A: Collapse is a main feature of von Neumann's theory of quantum measurement. In the Bohm interpretation, a wave does not collapse; instead, a measurement produces what Bohm called "empty channels" consisting of portions of the wave that no longer affect the particle. This conforms to the principle of decoherence, where a quantum system interacts with its environment to give the appearance of wavefunction collapse. The Bohm interpretation does not require a boundary between a quantum system and its classical environment.

Q: The Bohm interpretation involves reverse-engineering of quantum potentials and trajectories from standard QM. Diagrams in Bohm's book are constructed by forming contours on standard QM interference patterns and are not calculated from his "mathematical" formulation. Recent experiments with photons arXiv:quant-ph/0206196 v1 28 Jun 2002 favor standard QM over Bohm's trajectories.

A: The Bohm interpretation takes the Schrödinger equation even more seriously than does the conventional interpretation. In the Bohm interpretation, the quantum potential is a quantity derived from the Schrödinger equation, not a fundamental quantity. Thus, the interference patterns in the Bohm interpretation are identical to those in the conventional interpretation. As shown in [5] and [6], the experiments cited above only disprove a misinterpretation of the Bohm interpretation, not the Bohm interpretation itself.

Q: Hugh Everett says that Bohm's particles are not observable entities, but surely they are - what hits the detectors and causes flashes?

A: Both Everett and Bohm treat the wavefunction as a complex-valued but real field. Everett's Many-worlds interpretation is an attempt to demonstrate that the wavefunction alone is sufficient to account for all our observations. When we see the particle detectors flash or hear the click of a Geiger counter or whatever then Everett interprets this as our wavefunction responding to changes in the detector's wavefunction, which is responding in turn to the passage of another wavefunction (which we think of as a "particle", but is actually just another wave-packet). But no particle in the Bohm sense of having a defined position and velocity is involved. For this reason Everett sometimes referred to his approach as the "wave interpretation". Talking of Bohm's approach, Everett says:

“

Our main criticism of this view is on the grounds of simplicity - if one desires to hold the view that

ψ

is a real field then the associated particle is superfluous since, as we have endeavored to illustrate, the pure wave theory is itself satisfactory.^[15]

”

In this view, then, the Bohm particles are unobservable entities, similar to and equally as unnecessary as, for example, the luminiferous ether was found to be unnecessary in special relativity. We can remove the particles from Bohm's theory and still account for all our observations. The unobservability of the "hidden particles" stems from an asymmetry in the causal structure of the theory; the wavefunction influences the position and velocity of the hidden variables (i.e. the particles are influenced by a "force" exerted by the wavefunction), but the hidden variables do not influence the time development of the wavefunction (i.e. there is no analogue of Newton's third law -- the particles do not react back onto the wavefunction) Thus the particles do not make their presence known in any way; as the theory says, they are hidden.

[edit] See also

Wikiversity has learning materials about Making sense of quantum mechanics

[edit] References

^ Solvay Conference, 1928, Electrons et Photons: Rapports et Descussions du Cinquieme Conseil de Physique tenu a Bruxelles du 24 au 29 October 1927 sous les auspices de l'Institut International Physique Solvay
^ G. Brida, E. Cagliero, G. Falzetta, M.Genovese, M. Gramegna, C. Novero, A first experimental test of de Broglie-Bohm theory against standard quantum mechanics, J.Phys.B.At.Mol.Opt.Phys. 35 (2002) 4751 [1]
^ See Holland 1993, Chapter 8
^ Silverman and R. Huff, Ann. Phys. vol. 16, page 288 (1961), see also the discussion in Silverman 1993, section 3.3
^ Einstein, Podolsky, Rosen Can Quantum Mechanical Description of Physical Reality Be Considered Complete? Phys. Rev. 47, 777 (1935).
^ Bell, John S, Speakable and Unspeakable in Quantum Mechanics, Cambridge University Press 1987.
^ Bell, John S, Speakable and Unspeakable in Quantum Mechanics, Cambridge University Press 1987.
^ Solvay Conference, 1928, Electrons et Photons: Rapports et Descussions du Cinquieme Conseil de Physique tenu a Bruxelles du 24 au 29 October 1927 sous les auspices de l'Institut International Physique Solvay
^ von Neumann J. 1932 Mathematische Grundlagen der Quantenmechanik
^ See section VI of Everett's thesis: The Theory of the Universal Wave Function, pp 3-140 of Bryce Seligman DeWitt, R. Neill Graham, eds, The Many-Worlds Interpretation of Quantum Mechanics, Princeton Series in Physics, Princeton University Press (1973), ISBN 0-691-08131-X
^ Everett FAQ
^ Hyman, Ross et al Bohmian mechanics with discrete operators, J. Phys. A: Math. Gen. 37 L547-L558, 2004
^ Paul Davies, J R Brown, The Ghost in the Atom, ISBN 0-521-31316-3
^ J. S. Bell, On the Einstein Podolsky Rosen Paradox, Physics 1, 195 (1964)
^ See section VI of Everett's thesis: The Theory of the Universal Wave Function, pp 3-140 of Bryce Seligman DeWitt, R. Neill Graham, eds, The Many-Worlds Interpretation of Quantum Mechanics, Princeton Series in Physics, Princeton University Press (1973), ISBN 0-691-08131-X

Albert, David Z. (May 1994). "Bohm's Alternative to Quantum Mechanics". Scientific American.
Barbosa, G. D.; N. Pinto-Neto (2004). "A Bohmian Interpretation for Noncommutative Scalar Field Theory and Quantum Mechanics". Physical Review D 69: 065014. doi:10.1103/PhysRevD.69.065014. arΧiv:hep-th/0304105.
Bohm, David (1952). "A Suggested Interpretation of the Quantum Theory in Terms of "Hidden Variables" I". Physical Review 85: 166–179. doi:10.1103/PhysRev.85.166.
Bohm, David (1952). "A Suggested Interpretation of the Quantum Theory in Terms of "Hidden Variables", II". Physical Review 85: 180–193. doi:10.1103/PhysRev.85.180.
Bohm, David (1990). "A new theory of the relationship of mind and matter". Philosophical Psychology 3 (2): 271–286. doi:10.1080/09515089008573004. http://members.aol.com/Mszlazak/BOHM.html.
Bohm, David; B.J. Hiley (1993). The Undivided Universe: An ontological interpretation of quantum theory. London: Routledge. ISBN 0-415-12185-X.
Durr, Detlef; Sheldon Goldstein, Roderich Tumulka and Nino Zangh (December 2004) (PDF). Bohmian Mechanics. http://www.math.rutgers.edu/~oldstein/papers/bohmech.pdf.
Goldstein, Sheldon (2001). "Bohmian Mechanics". Stanford Encyclopedia of Philosophy. http://plato.stanford.edu/entries/qm-bohm/.
Hall, Michael J.W. (2004). "Incompleteness of trajectory-based interpretations of quantum mechanics". Journal of Physics a Mathematical and General 37: 9549. doi:10.1088/0305-4470/37/40/015. arΧiv:quant-ph/0406054. (Demonstrates incompleteness of the Bohm interpretation in the face of fractal, differentialble-nowhere wave functions.)
Holland, Peter R. (1993). The Quantum Theory of Motion : An Account of the de Broglie-Bohm Causal Interpretation of Quantum Mechanics. Cambridge: Cambridge University Press. ISBN 0-521-48543-6.
Nikolic, H. (2004). "Relativistic quantum mechanics and the Bohmian interpretation". Foundations of Physics Letters 18: 549. doi:10.1007/s10702-005-1128-1. arΧiv:quant-ph/0406173.
Passon, Oliver (2004). Why isn't every physicist a Bohmian?. arΧiv:quant-ph/0412119.
Sanz, A. S.; F. Borondo (2003). "A Bohmian view on quantum decoherence". The European Physical Journal D 44: 319. doi:10.1140/epjd/e2007-00191-8. arΧiv:quant-ph/0310096.
Sanz, A.S. (2005). "A Bohmian approach to quantum fractals". J. Phys. A: Math. Gen. 38: 319. doi:10.1088/0305-4470/38/26/013. (Describes a Bohmian resolution to the dilemma posed by non-differentiable wave functions.)
Silverman, Mark P. (1993). And Yet It Moves: Strange Systems and Subtle Questions in Physics. Cambridge: Cambridge University Press. ISBN 0-521-44631-7.
Streater, Ray F. (2003). "Bohmian mechanics is a "lost cause"". http://www.mth.kcl.ac.uk/~streater/lostcauses.html#XI. Retrieved on 2006-06-25.
Valentini, Antony; Hans Westman (2004). Dynamical Origin of Quantum Probabilities. arΧiv:quant-ph/0403034.
Bohmian mechanics on arxiv.org

[edit] External links

"Bohmian Mechanics" (Stanford Encyclopedia of Philosophy)
"Pilot waves, Bohmian metaphysics, and the foundations of quantum mechanics", lecture course on Bohm interpretation by Mike Towler, Cambridge University.

[0] Solvay Conference, 1928, Electrons et Photons: Rapports et Descussions du Cinquieme Conseil de Physique tenu a Bruxelles du 24 au 29 October 1927 sous les auspices de l'Institut International Physique Solvay

[1] G. Brida, E. Cagliero, G. Falzetta, M.Genovese, M. Gramegna, C. Novero, A first experimental test of de Broglie-Bohm theory against standard quantum mechanics, J.Phys.B.At.Mol.Opt.Phys. 35 (2002) 4751 [1]

[2] See Holland 1993, Chapter 8

[3] Silverman and R. Huff, Ann. Phys. vol. 16, page 288 (1961), see also the discussion in Silverman 1993, section 3.3

[4] Einstein, Podolsky, Rosen Can Quantum Mechanical Description of Physical Reality Be Considered Complete? Phys. Rev. 47, 777 (1935).

[5] Bell, John S, Speakable and Unspeakable in Quantum Mechanics, Cambridge University Press 1987.

[6] Bell, John S, Speakable and Unspeakable in Quantum Mechanics, Cambridge University Press 1987.

[7] Solvay Conference, 1928, Electrons et Photons: Rapports et Descussions du Cinquieme Conseil de Physique tenu a Bruxelles du 24 au 29 October 1927 sous les auspices de l'Institut International Physique Solvay

[8] von Neumann J. 1932 Mathematische Grundlagen der Quantenmechanik

[9] See section VI of Everett's thesis: The Theory of the Universal Wave Function, pp 3-140 of Bryce Seligman DeWitt, R. Neill Graham, eds, The Many-Worlds Interpretation of Quantum Mechanics, Princeton Series in Physics, Princeton University Press (1973), ISBN 0-691-08131-X

[10] Everett FAQ

[11] Hyman, Ross et al Bohmian mechanics with discrete operators, J. Phys. A: Math. Gen. 37 L547-L558, 2004

[12] Paul Davies, J R Brown, The Ghost in the Atom, ISBN 0-521-31316-3

[Bell_1964-13] J. S. Bell, On the Einstein Podolsky Rosen Paradox, Physics 1, 195 (1964)

[14] See section VI of Everett's thesis: The Theory of the Universal Wave Function, pp 3-140 of Bryce Seligman DeWitt, R. Neill Graham, eds, The Many-Worlds Interpretation of Quantum Mechanics, Princeton Series in Physics, Princeton University Press (1973), ISBN 0-691-08131-X

[1]

[2]

[3]

[4]

[5]

[6]

[7]

[8]

[9]

[10]

[11]

[12]

[13]

[14]

[15]

Bohm interpretation

From Wikipedia, the free encyclopedia

Contents

[edit] The theory

[edit] Principles

[edit] Name and evolution

[edit] Results

[edit] Reformulating the Schrödinger equation

[edit] One-particle formalism

[edit] Many-particle formalism

[edit] Comparison with experimental data

[edit] Understanding quantum mechanics

[edit] Indeterminism in the Bohm Interpretation.

[edit] Heisenberg's uncertainty principle

[edit] Two-slit experiment

[edit] Measuring spin and polarization

[edit] The Einstein-Podolsky-Rosen paradox

[edit] History

[edit] Extensions

[edit] Exploiting Nonlocality

[edit] Isomorphism to the many worlds interpretation

[edit] Quantum trajectory method

[edit] Quantum chaos

[edit] Other extensions

[edit] Frequently asked questions

[edit] See also

[edit] References

[edit] External links

Views

Personal tools

Navigation

Search

Interaction

Toolbox

Languages